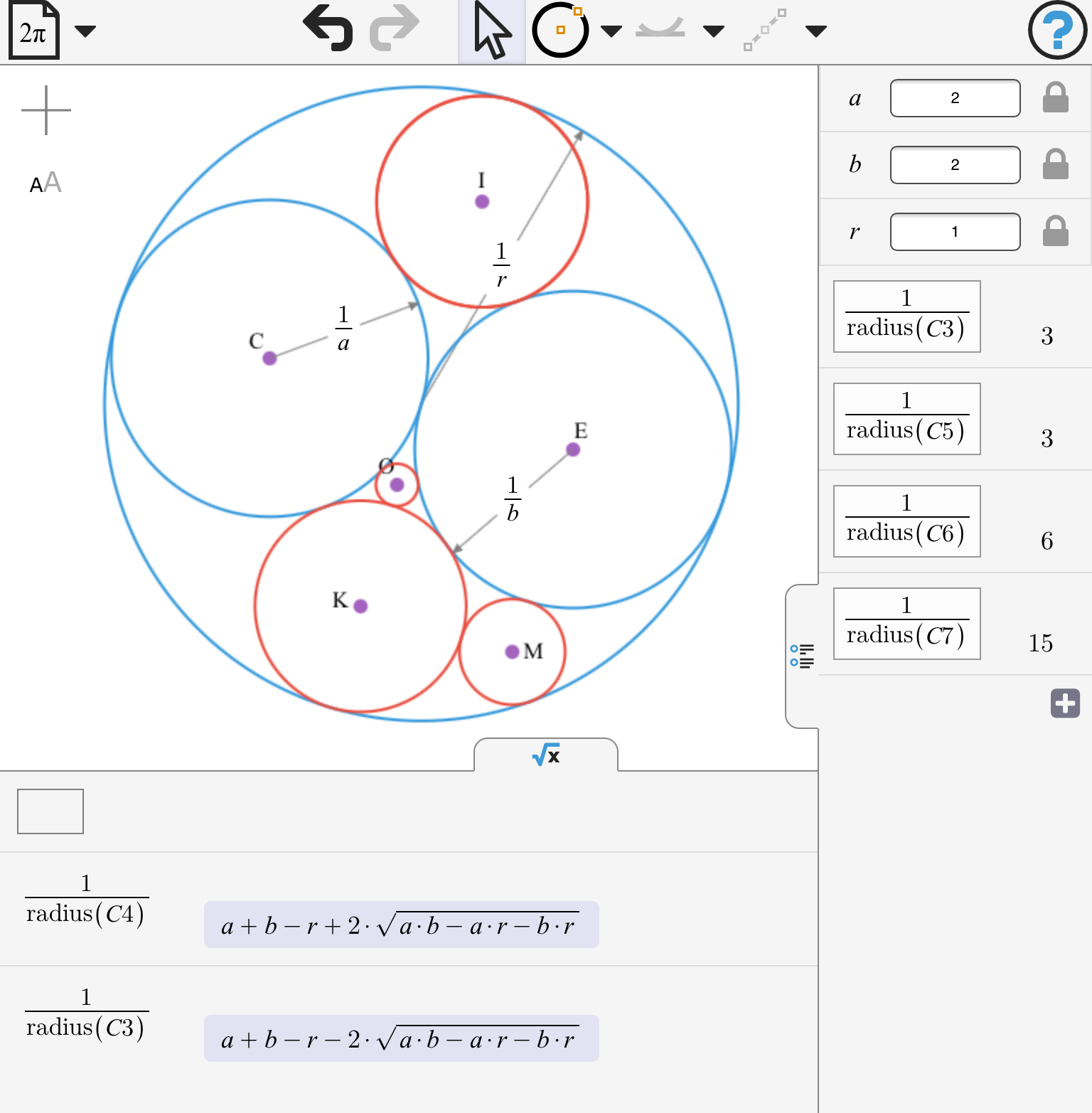
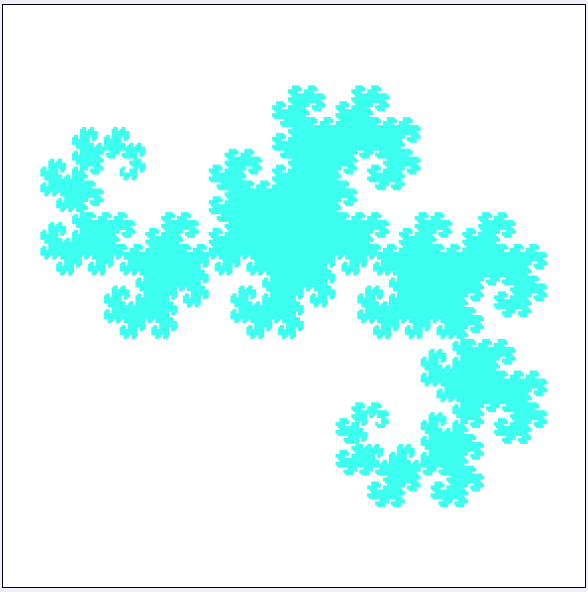
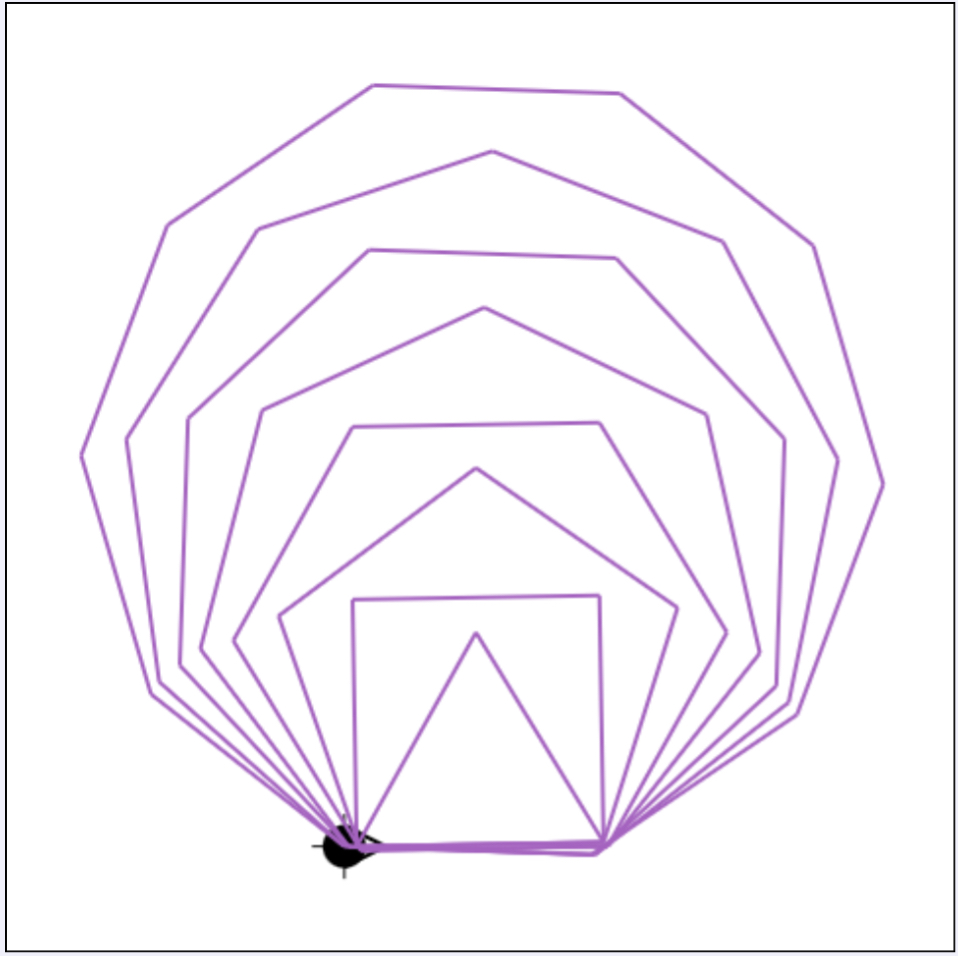
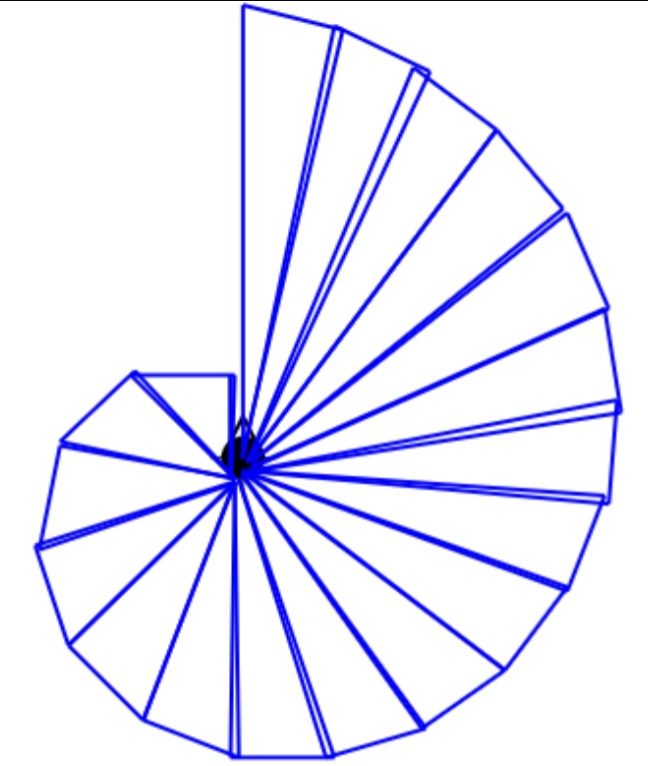
©2021 Compass Learning Technologies → GXWeb Showcase → GXWeb Jigsaws → GXWeb Mathematics Explorer
GXWeb Mathematics Explorer
Saltire Software, home of Geometry Expressions and GXWeb
Symbolic computations on this page use the Nerdamer Symbolic JavaScript to complement the in-built computer algebra system of GXWeb.
The beautiful mathematics you see on this page is made possible using MathQuill and MathJax.
Learn How: Create Your Own GXWeb Tasks (like this one!)
Learn How: Add Beautiful Mathematics to your Web Page
Introduction
About the MathBoxes...