GXWeb Fractured Fractions Collection
Saltire Software, home of Geometry Expressions and GXWeb
Symbolic computations on this page use Nerdamer Symbolic JavaScript to complement the in-built CAS of GXWeb
An Introduction to Continued Fractions by Dr Ron Knott
Chaos in Numberland: The secret life of continued fractions by John D. Barrow
Explore Bill Gosper (1972): Continued Fraction Arithmetic
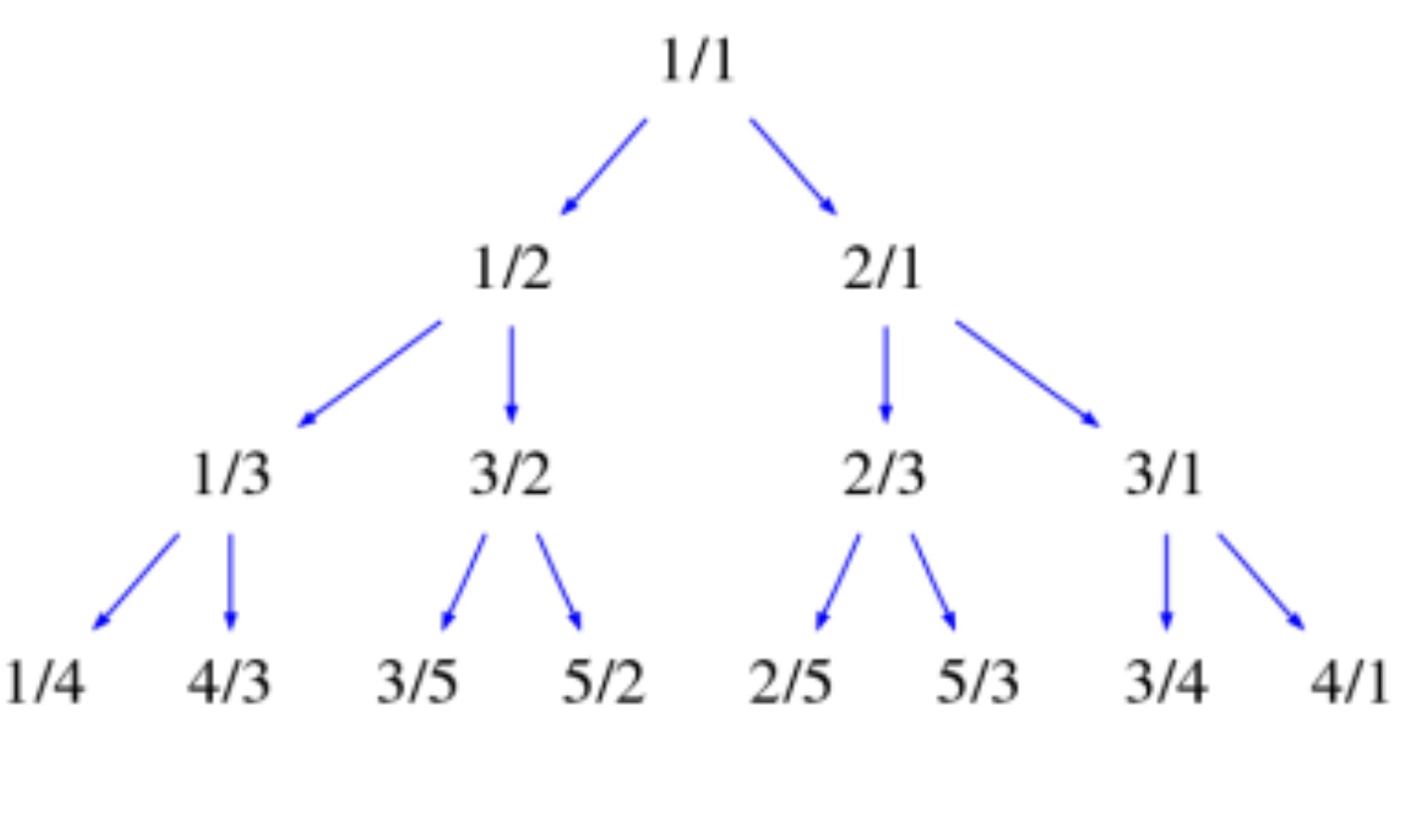
Calkin and Wilf (1999): Recounting the Rationals
Bruce Bates (2014): The Stern-Brocot Continued Fraction
Bates, B., Bunder, M. and Tognetti, K. (2010): Linking the Calkin–Wilf and Stern–Brocot trees
With thanks to the late Dr Keith Tognetti for pointing me in the direction of continued fractions many years ago and igniting in me a life-long passion, and to Dr Bruce Bates (both of the University of Wollongong) for revealing to us all the beautiful Stern-Brocot Continued Fraction. With colleague Dr Martin Bunder they have brought to light many wonderful and often surprising connections between binary trees and continued fractions.
Welcome to the Fractured Fractions Collection!
Try the Continued Fraction JigSaw! Just rearrange the squares to fill the given rectangle, and then press INPUT (or the JIGSAW button again) to enter your answer.
Then go on and explore some of the wonderful connections between continued fractions and some surprising and important corners of mathematics. Did you know, for example, that fractions grow on trees?
Then dig deeper into Continued Fractions.
|
Drag point P or use the INPUT button to explore App generated by GXWeb \[ \frac{10}{7} = [1,2,3]\] \[ ⇒ 1 + \frac{1}{2 + \frac{1}{3}}\] \[ \approx 1.4285714286\] |
Introduction
If you have not come across continued fractions in your mathematical travels, then it is high time you did!
Every real number, rational and irrational, can be represented as a continued fraction. While normal fractions can only represent rational numbers, continued fractions are different - full of surprising patterns and relationships.
Not surprisingly, rational numbers produce finite continued fractions, while irrationals become infinite continued fractions.
107=1+37=1+173=1+12+13
Unlike irrational decimals, however, even irrational continued fractions can be predictable and are an ideal way to calculate approximate values - as accurately as you like!
Continued fractions have many practical applications but one of the most important lies in their ability to offer VERY good approximations to irrational numbers - the more convergents, the better the approximation. Indeed, each convergent gives the Best Approximation of the First Kind (look that up!) AND if a large value turns up in the list, then cutting the continued fraction just before that large value gives an extremely accurate approximation!
Study the examples which follow and see what you notice.
Some Examples
The Golden Ratio (or Golden Mean) (1 + sqrt(5))/2: 1+√52
ϕ≈1+11+11+11+11+⋯
Tap above or enter \phi in the MathBox below and press the f(x) button), OR for another approach, think of this continued fraction as x=1+1x=>x2=x+1
EXPLORE: Can you see why 1+√2=(2+√82) is called the silver mean and 3+√132 is the bronze mean? What would be their quadratic equations? Any suggestions for copper, nickel, aluminium or other metallic means?
Sqrt(2) ⇒ √2≈1+12+12+12+12+⋯
Tap above or enter \sqrt(2) in the MathBox below and press the f(x) button) - or try x=1+11+x=>x2=2
EXPLORE: Can you find the quadratic equation form for √(3) or √(5)?
Pi ⇒ π≈3+17+115+11+1292+⋯
(Tap above or enter \pi in the MathBox below and press the f(x) button) (but no closed form equation for this one!)
Continued fractions do come in more than one flavour.
In most cases, we deal with SIMPLE continued fractions, for which the numerators are all equal to 1, as shown above.
The famous Indian mathematician, Srinivasa Ramanujan, along with Euler, Gauss and other greats over many years have been fond of far more interesting varieties - visit GXWeb Fractured Functions to learn more about these amazing creatures.
|
π≈41+123+225+327+429+5211+6213+⋯ |
|
e≈3−14−25−36−47−58−69+⋯ |
Continued fractions continue to be a source of research in many branches of mathematics. Use the tools available here to explore these wonderful mathematical opportunities!
You might notice that while simple continued fractions give predictable values for only a limited range of real numbers (the so-called Quadratic Irrationals), examples like these show that even the most transcendental of numbers can be expressed in predictable form. Observe, below, that continued fractions can also be expressed as the product of matrices!
Mathematics has been described as a search for patterns and relationships. What patterns and relationships did you find in these few examples? Use the tools which follow to explore your ideas further, and take a few moments to answer the questions that follow.
Once you have finished the questions, feel free to come back and continue to play with and learn more about these extraordinary numbers. They can be a gateway to interesting and important topics in mathematics, nature and computing.
Explore the Magic Table
Introduction: What is a Magic Table?
To convert a real number to a continued fraction is easy! Try this yourself?
But what if you want to go in the other direction? If you have a continued fraction, and would like to know the real number that it represents?
For some rational numbers (and approximations of irrationals), this is (relatively) easy, since the continued fraction is finite. For most, though, it can be quite arduous.
The most commonly used method involves starting from the bottom and working upwards. This approach, however, does not reveal the successive approximations, the convergents, which in many cases may be just as useful as the final result.
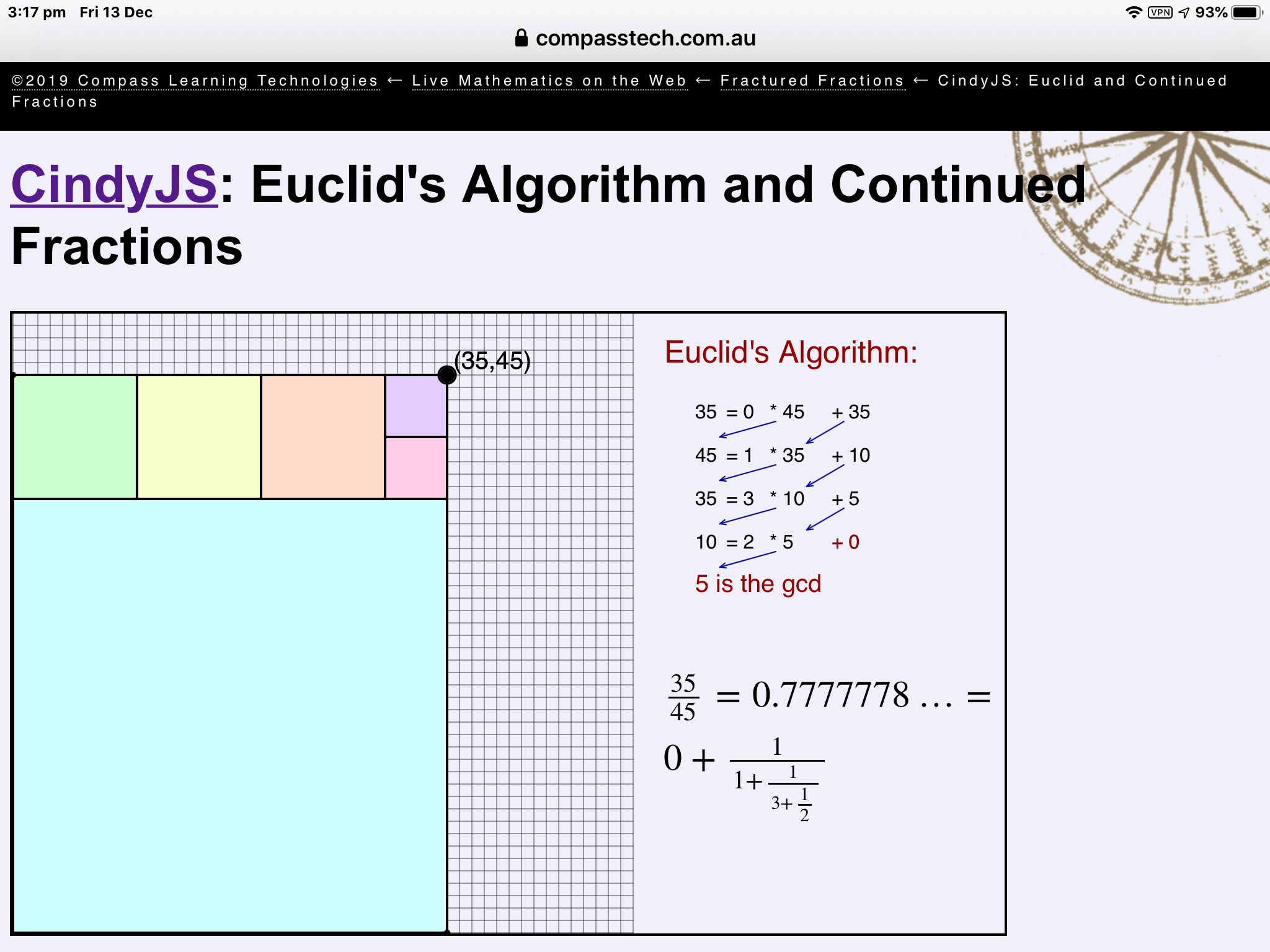
\[ \frac{10}{7}\]\[= 1+\frac{3}{7}\]\[= 1 + \frac{1}{\frac{7}{3}}\]\[= 1 + \cfrac{1}{2 + \cfrac{1}{3}} \]\[= [1,2,3]\]
\[ [1,2,3]\]\[= 1 + \cfrac{1}{2 + \cfrac{1}{3}} \]\[= 1 + \frac{1}{\frac{7}{3}}\]\[= 1+\frac{3}{7} \]\[= \frac{10}{7} \]
Alternatively, you might try the Magic Table method!
The magic table 1, 2 (first described by Gosper (1972) - credit for the name goes to Associate Professor Terry Gagen of the University of Sydney) offers an efficient and (relatively) simple way to generate the convergents of a continued fraction - it can even be used, in 1 or 2 dimensions, on linear fractional transformations of continued fractions and, in 3 dimensions, forms the basis for Gosper's algorithm for continued fraction arithmetic.
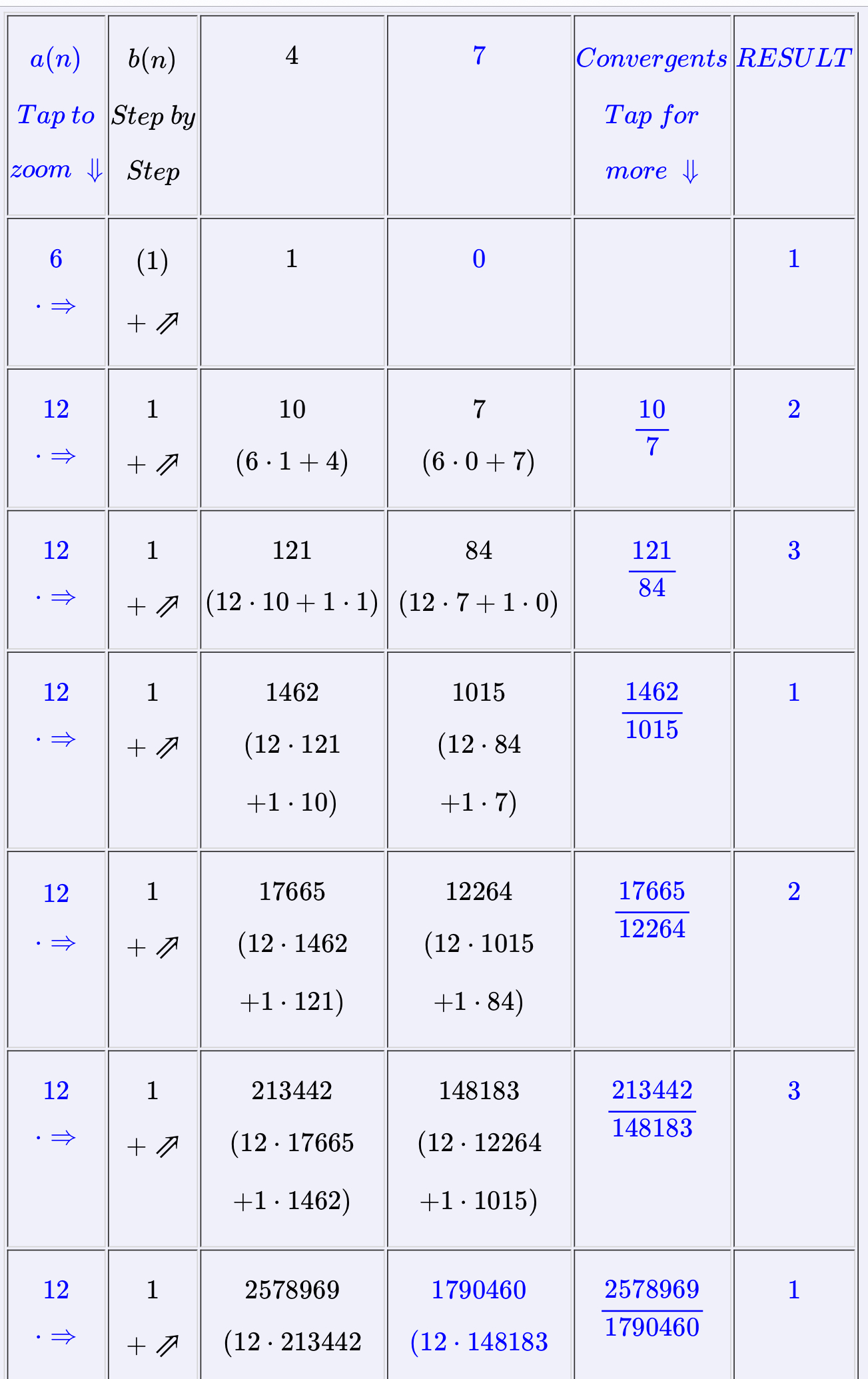
For example, the continued fraction of
\[\sqrt(37) => \]\[a(n) = [6,12,12,12,12,12,12,...]\]\[b(n) = [1,1,1,1,1,1,1...]\] \[6 + \cfrac{1}{12 + \cfrac{1}{12 + \cfrac{1}{12 + \cfrac{1}{12 + \cfrac{1}{12 + \cfrac{1}{12 + \cfrac{1}{12... }}}}}}}\]Using the approaches described here is much simpler than, for example, matrix methods to calculate a result such as
\[\frac{\sqrt{37} + 4}{7} = \begin{bmatrix} 1 && 4 \\ 0 && 7 \end{bmatrix} \cdot \sqrt(37) = \begin{bmatrix} 1 && 4 \\ 0 && 7 \end{bmatrix} \cdot \begin{bmatrix} \sqrt(37) && 0 \\ 0 && 1 \end{bmatrix} \]where the linear fractional transformation is the matrix
\[\begin{bmatrix} 1 && 4 \\ 0 && 7 \end{bmatrix} \ and \ \begin{bmatrix} 1 && 0 \\ 0 && 1 \end{bmatrix}\]represents the identity matrix.
\[...\] \[12\] \[12\] \[12\] \[12\] \[12\] \[6\] \[a(n)\] \[...\] \[1\] \[1\] \[1\] \[1\] \[1\] \[(1)\] \[b(n)\] \[...\] \[213442\] \[17665\] \[1462\] \[121\] \[10\] \[1\] \[4\] \[...\] \[148183\] \[12264\] \[1015\] \[84\] \[7\] \[0\] \[7\]
Introducing the Magic Table: Dimension 1
Gosper's original (dimension 1) version of the magic table was presented horizontally, reading from right to left. A simplified form (which delivers each convergent in turn) might be represented as shown above.
Note that Gosper dealt only with simple continued fractions for which all b(n) (numerator) terms may be assumed to be 1. We go a step further here, adding a b(n) row to support general continued fractions.
Can you see how the pairs of numbers below the continued fraction terms are calculated? (Notice that the first convergent is calculated directly from the terms of the linear fractional transformation.)
Tap on these pairs to see, or tap on the red continued fraction terms - from right to left - to see Gosper's Magic Table in action!
Introducing the Magic Table: Dimension 2
More importantly, Gosper was not really interested in simply outputting the convergents of continued fractions.
His real goal was to take a continued fraction, operate on it in some way - initially, using a linear fractional transformation, but leading to arithmetic operations with another continued fraction - and output the result directly as a continued fraction: true continued fraction arithmetic!
For this, he needed more dimensions for his magic table!
Teasing Out the Resultant Continued Fraction
It should be noted that drawing out each term of the resultant continued fraction is not always a straightforward operation.
Consider the first TWO convergents of
\[\frac{\sqrt{37} + 4}{7} = \begin{bmatrix} 1 && 4 \\ 0 && 7 \end{bmatrix} \cdot \sqrt(37)\] \[=> \frac{10}{7} \ and \ \frac{121}{84}\]Taking the floor or integer part of both delivers the same result (\(|\frac{10}{7}| = |\frac{121}{84}| = 1\)), so we can be confident in the first term of our resultant continued fraction.
To continue, we need the dimension 2 table, since we now move one step down and one step to the left (in the table above, or to the right in the table below). The next two convergents to consider are \(\frac{84}{37}\) and \(\frac{1015}{447}\). If the floor values of both agree (which they do in this case), we continue this process, moving down and horizontally forward.
If NOT, then we simply move one step horizontally forward, and try the next pair of convergents, until we find two that agree, and this process gives each subsequent term of our resultant continued fraction.
For a more interesting example, consider Gosper's case of \(e\) with linear fractional transformation, [1,-1,1,1]:
\[tanh(\frac{1}{2}) = \frac{e-1}{e+1}\]Here we see that the first convergent pair (\(\frac{1}{3}\) and \(\frac{2}{4}\)) both deliver floor values of 0. The second pair (\(\frac{11}{5}\) and \(\frac{4}{2}\)) give us the next resultant term of 2.
Subsequently, though, \(|\frac{5}{1}| = 5\) and \(|\frac{7}{1}| = 7\). We must continue to the next terms, \(\frac{12}{2}\) and (with a little calculation) \(\frac{55}{9}\) (\(4 \cdot 12 + 1 \cdot 7 = 55\) and \(4 \cdot 2 + 1 \cdot 1 = 9\)) to confirm the floor result of 6.
The floor values of pairs of adjacent convergents must differ by no more than 1 to deliver the next term of the resultant continued fraction.
For ease of presentation, in our versions, the terms are displayed vertically, with the terms of the linear fractional transformation found at the top of the numerator and denominator columns (rotated -90°).
Step by Step
Tap to view:
Simple Convergent Form 1
Simple Convergent Form 2
Gosper Magic Table 1
Gosper Magic Table 2
Gosper Magic Table 3
Gosper Magic Table 4
Gosper Magic Table 5
In its simplest form, Gosper's Magic Table initially offers a convenient way to generate the convergents for a given continued fraction, along with a linear fractional transformation, expressed as a 2x2 matrix.
(Tap for an example) ⇑
General Continued Fractions: Some Examples
Note that the Magic Table presented here represents an extension of Gosper's method, supporting general continued fractions in addition to simple ones. For example, did you know that
\[\pi \approx 0 + \cfrac{4}{1 + \cfrac{1}{3 + \cfrac{4}{5 + \cfrac{9}{7 + \cfrac{16}{9 + \cfrac{25}{11 + \cfrac{36}{13 + \cfrac{49}{15 + \cfrac{64}{17 + \cfrac{81}{19...}}}}}}}}}}\]\[a(n) = [0,1,3,5,7,9,11,13,15,17,19]\]\[b(n) = [4,1,4,9,16,25,36,49,64,81]\]
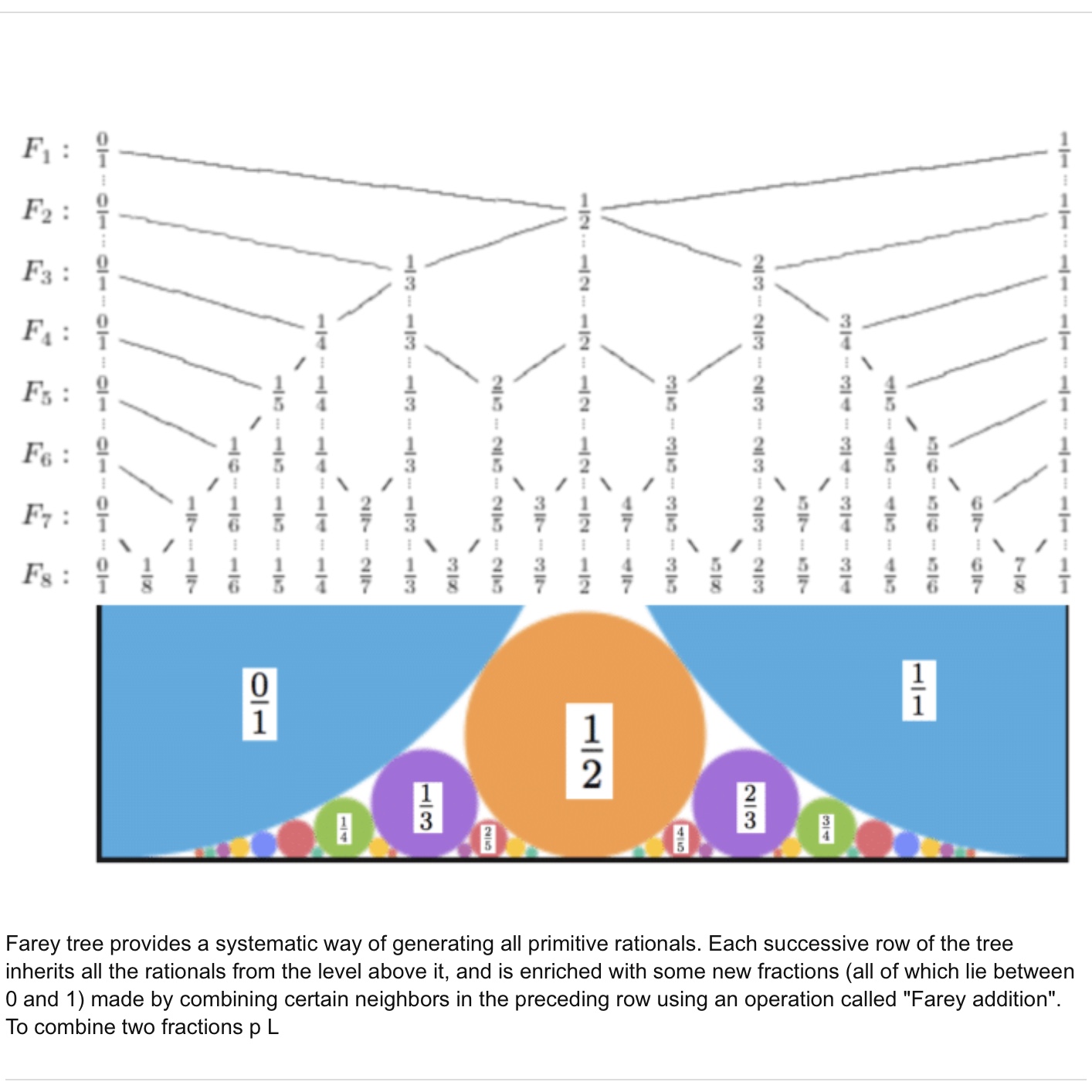
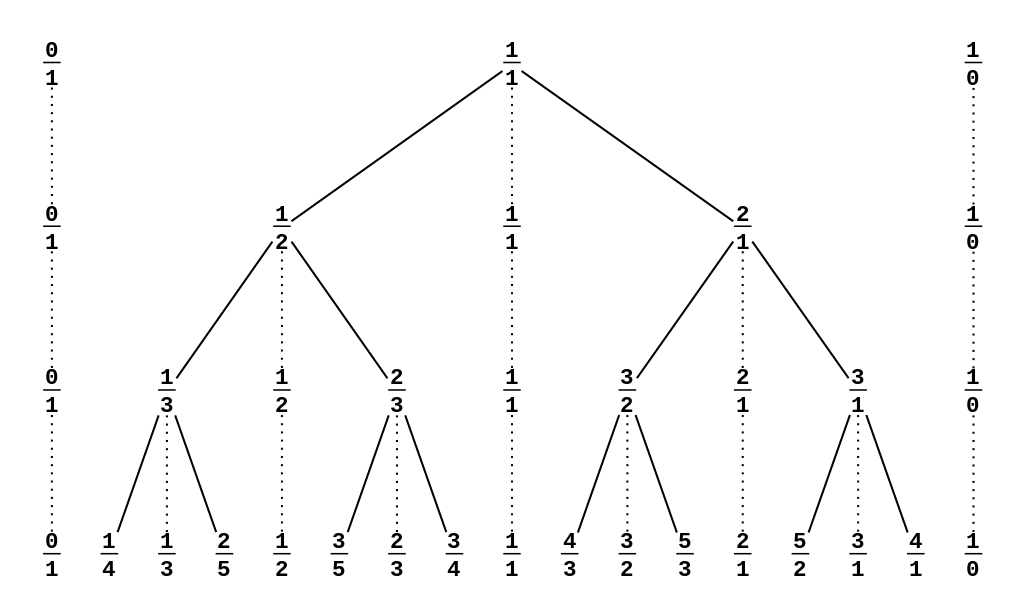
And what about the Farey and Stern-Brocot Continued Fractions: if you wanted to start a list of every rational number, then these amazing continued fractions are perfect for that!
\[0 + \cfrac{1}{3 - \cfrac{1}{1 - \cfrac{1}{3 - \cfrac{1}{1}}}} = 0 + \cfrac{1}{3 + \cfrac{1}{-1 + \cfrac{1}{3 + \cfrac{1}{-1}}}}\] \[⇒ [0,\frac{1}{3},\frac{1}{2},\frac{2}{3},1]\]
Farey Sequence Level 3:mtable([[0,3,-1,3,-1],[1,1,1,1,1]],[1,0,0,1])
OR mtable([[0,3,1,3,1],[1,-1,-1,-1,-1]],[1,0,0,1])
\[0 + \cfrac{1}{4 - \cfrac{1}{1 - \cfrac{1}{3 - \cfrac{1}{1 - \cfrac{1}{5 - \cfrac{1}{1 - \cfrac{1}{3 - \cfrac{1}{1}}}}}}}}\] \[⇒ [0,\frac{1}{4}, \frac{1}{3}, \frac{2}{5},\frac{1}{2}, \frac{3}{5}, \frac{3}{4}, \frac{2}{3},1]\] Farey Sequence Level 4:
mtable([[0,4,-1,3,-1,5,-1,3,-1],[1,1,1,1,1,1,1,1,1]],[1,0,0,1])
OR mtable([[0,4,1,3,1,5,1,3,1],[1,-1,-1,-1,-1,-1,-1,-1,-1]],[1,0,0,1])
\[0 + \cfrac{1}{3 - \cfrac{1}{1 - \cfrac{1}{3 - \cfrac{1}{1 - \cfrac{1}{5 - \cfrac{1}{1 - \cfrac{1}{3 - \cfrac{1}{1}}}}}}}}\] \[⇒ [0,\frac{1}{3},\frac{1}{2},\frac{2}{3},1,\frac{3}{2},\frac{2}{1}, \frac{3}{1},\frac{1}{0}]\] Stern-Brocot Sequence Level 3:
mtable([[0,3,-1,3,-1,5,-1,3,-1],[1,1,1,1,1,1,1,1,1]],[1,0,0,1])
OR mtable([[0,3,1,3,1,5,1,3],[1,-1,-1,-1,-1,-1,-1,-1]],[1,0,0,1])
Stern-Brocot Sequence Level 4:
\[0 + \cfrac{1}{4 - \cfrac{1}{1 - \cfrac{1}{3 - \cfrac{1}{1 - \cfrac{1}{5 - \cfrac{1}{1 - \cfrac{1}{3 - \cfrac{1}{1 - \cfrac{1}{7 - \cfrac{1}{1 - \cfrac{1}{3 - \cfrac{1}{1 - \cfrac{1}{5 - \cfrac{1}{1 - \cfrac{1}{3 - \cfrac{1}{1}}}}}}}}}}}}}}}}\] \[⇒ [0,\frac{1}{4}, \frac{1}{3}, \frac{2}{5},\frac{1}{2}, \frac{3}{5}, \frac{3}{4}, \frac{2}{3},1, \frac{3}{2}, \frac{4}{3}, \frac{5}{3},\frac{2}{1}, \frac{5}{2}, \frac{3}{1}, \frac{4}{1}, \frac{1}{0}]\]mtable([[0,4,-1,3,-1,5,-1,3,-1,7,-1,3,-1,5,-1,3],[1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1]],[1,0,0,1],0,17)
OR mtable([[0,4,1,3,1,5,1,3,1,7,1,3,1,5,1,3],[1,-1,-1,-1,-1,-1,-1,-1,-1,-1,-1,-1,-1,-1,-1,-1,-1]],[1,0,0,1],0,17)
Try More Examples
Simple (Convergent)
Magic Table
Dimension 1Gosper
Magic Table
Dimension 2Enter mtable(), or
mtable(sqrt(37)), or
mtable(sqrt(37),[1,4,0,7]), or even
mtable(sqrt(37),[1,4,0,7],0,12)Try one of Gosper's examples:
mtable(sqrt(2),[0,2,-1,3],0,12)You can also enter arrays: mtable([1,2,3,4,5],[1,0,0,1],0,12) or
mtable([[1,2,3,4],[4,3,2,1],[1,0,0,1],0,12)Try mtable([[2,1,2,3,4,5,6,7,8,9,10],[1,1,2,3,4,5,6,7,8,9,10]],[1,0,0,1],0,12)
Enter gmt(), or
gmt(sqrt(37)), or
gmt(sqrt(37),[1,4,0,7]), or even
gmt(sqrt(37),[1,4,0,7],0,12)Try one of Gosper's examples:
gmt(sqrt(2),[0,2,-1,3],0,12)You can also enter arrays: gmt([1,2,3,4,5],[1,0,0,1],0,12) or
gmt([[1,2,3,4],[4,3,2,1],[1,0,0,1],0,12)Try gmt([[2,1,2,3,4,5,6,7,8,9,10],[1,1,2,3,4,5,6,7,8,9,10]],[1,0,0,1],0,12)
mtable([[0,1,3,5,7,9,11,13,15,17,19],[4,1,4,9,16,25,36,49,64,81,100]],[1,0,0,1],0,12).
Perhaps even compare
mtable([[4,2,2,2,2,2,2,17,294],[-1,-1,-1,-1,-1,-1,-1,-1,-1]],[1,0,0,1]) with
mtable([[4,-2,2,-2,2,-2,2,-17,294],[1,1,1,1,1,1,1,1,1]],[1,0,0,1])?
Build Your Own Continued Fraction
To convert a real number (n) to a continued fraction is easy!
Try this yourself?
Step 1: Take the integer part of the number - floor[n] or Math.floor(n): This is your first index (call it a0), and first convergent.
Step 2: Subtract the integer part to leave the decimal part - the tail. Tail=n−floor(n)
Step 3: Calculate the reciprocal of the tail (1Tail) - this should give a number greater than or equal to 1.
Step 4: Take the integer part of this reciprocal value (a1=floor(1Tail)): This is your next index - call it a1, and a0+1a1 the next convergent.
Step 5: Return to Step 2 and repeat the process...
But what if you want to go in the other direction? If you have a continued fraction, and would like to know the real number that it represents?
For rational numbers, this is easy, since the continued fraction is finite.
The most commonly used method involves starting from the bottom and working upwards.
Alternatively, try the Magic Table!
For most irrationals, finding the real number represented by a continued fraction is much harder, but not for quadratic irrationals, which are periodic.
Study the examples above for the golden ratio and the square root of 2. For any periodic continued fraction, we need to isolate the periodic part and use this to form a quadratic equation. For example...
[1,2,3,1,2,3,1,2,3...]=1+12+13+11+12+13+... x=1+12+13+1xMathematical ToolBox
About the MathBoxes...
Hint: When entering mathematical expressions in the math boxes below (f(x), g(x) and h(x)), use the space key to step out of fractions, powers, etc. On Android, begin entry by pressing Enter.
Type simple mathematical expressions and equations as you would normally enter these: for example, "x^2[space]-4x+3", and "2/3[space]". For more interesting elements, use Latex notation (prefix commands such as "sqrt" and "nthroot3" with a backslash (\)): for example: "\sqrt(2)[space][space]". Learn More?
|
f(x)
g(x)
h(x) |
|
a= -1 5 1 b= -1 1 5 c= -1 1 5
|
|
|
Continued Fraction Spreadsheet Explorer
This browser-based spreadsheet uses the handsontable JavaScript library.
WolframAlpha: CAS+
Sometimes, to deal with those stubborn, hard to reach problems, you need something stronger!
The powerful Wolfram Alpha online CAS engine will answer almost anything you care to ask - within reason! From the continued fraction of pi to Solve x^2=x+1 to the population of Australia!
GeoGebra offers another fast and accurate CAS alternative. Use the button above ⇑ for quick computation, or you may like to explore the full GeoGebra web app. ⇒