©2021 Compass Learning Technologies ← Live Mathematics on the Web ← GXWeb Showcase ← Continued Fraction Jigsaw →GXWeb Fractured Fractions
GXWeb Fractured Fractions
Saltire Software, home of Geometry Expressions and GXWeb
Download a Geometry Expressions Model for Fractured Fractions
Symbolic computations on this page use Nerdamer Symbolic JavaScript to complement the in-built CAS of GXWeb
An Introduction to Continued Fractions by Dr Ron Knott
Chaos in Numberland: The secret life of continued fractions by John D. Barrow
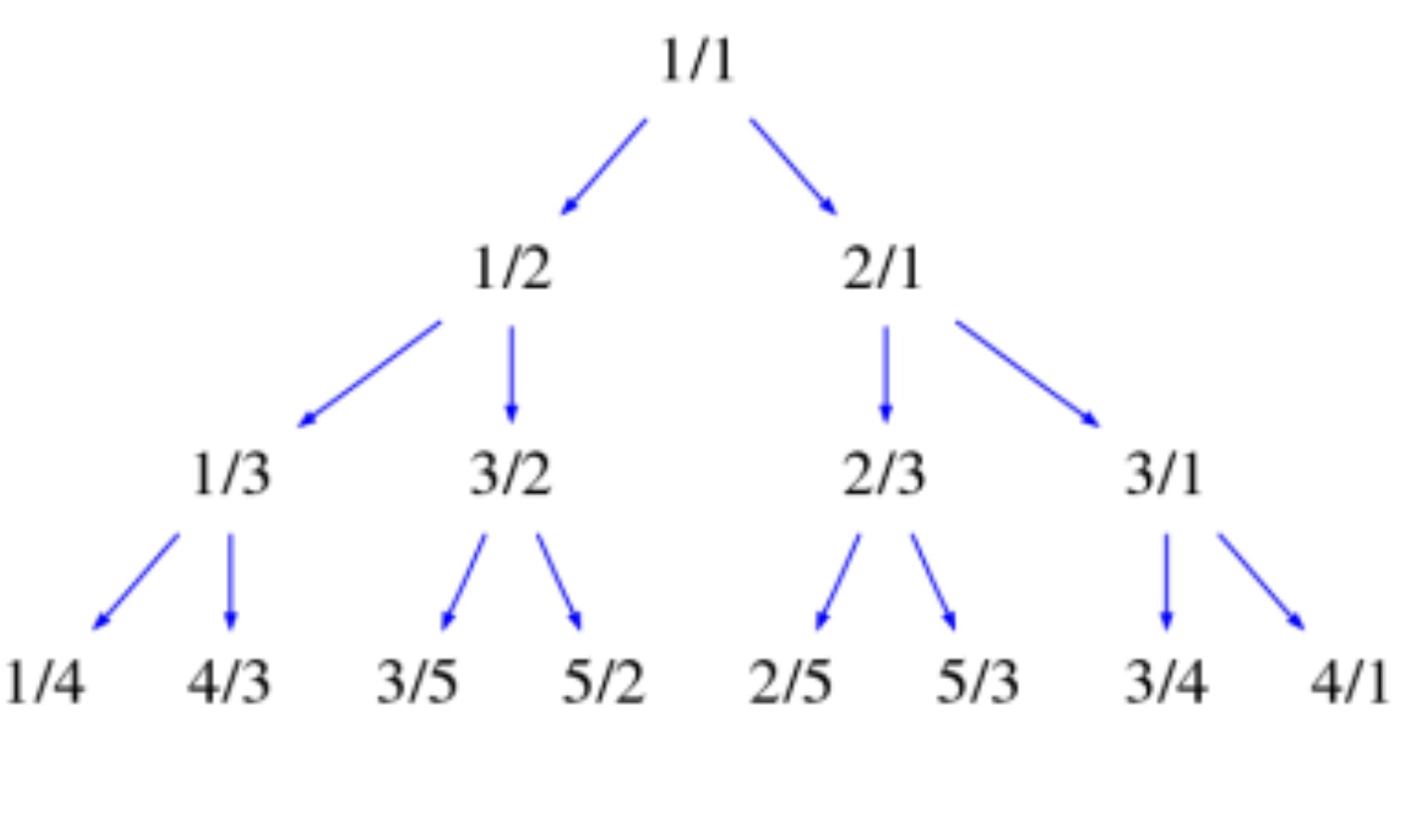
Bruce Bates (2014): The Stern-Brocot Continued Fraction
Bates, B., Bunder, M. and Tognetti, K. (2010): Linking the Calkin-Wilf and Stern-Brocot trees
With special thanks to the late Dr Keith Tognetti for pointing me in the direction of continued fractions many years ago and igniting in me a life-long passion, and to Dr Bruce Bates (both of the University of Wollongong) for revealing the beautiful Stern-Brocot Continued Fraction
Continue your continued fraction journey after this...
2. Try the Continued Fraction Jigsaw!
3. Explore the Continued Fraction Collection!
4. Take Continued Fractions to the Next Level with Fractured Functions.
Introduction
If you have not come across continued fractions in your mathematical travels, then it is high time you did!
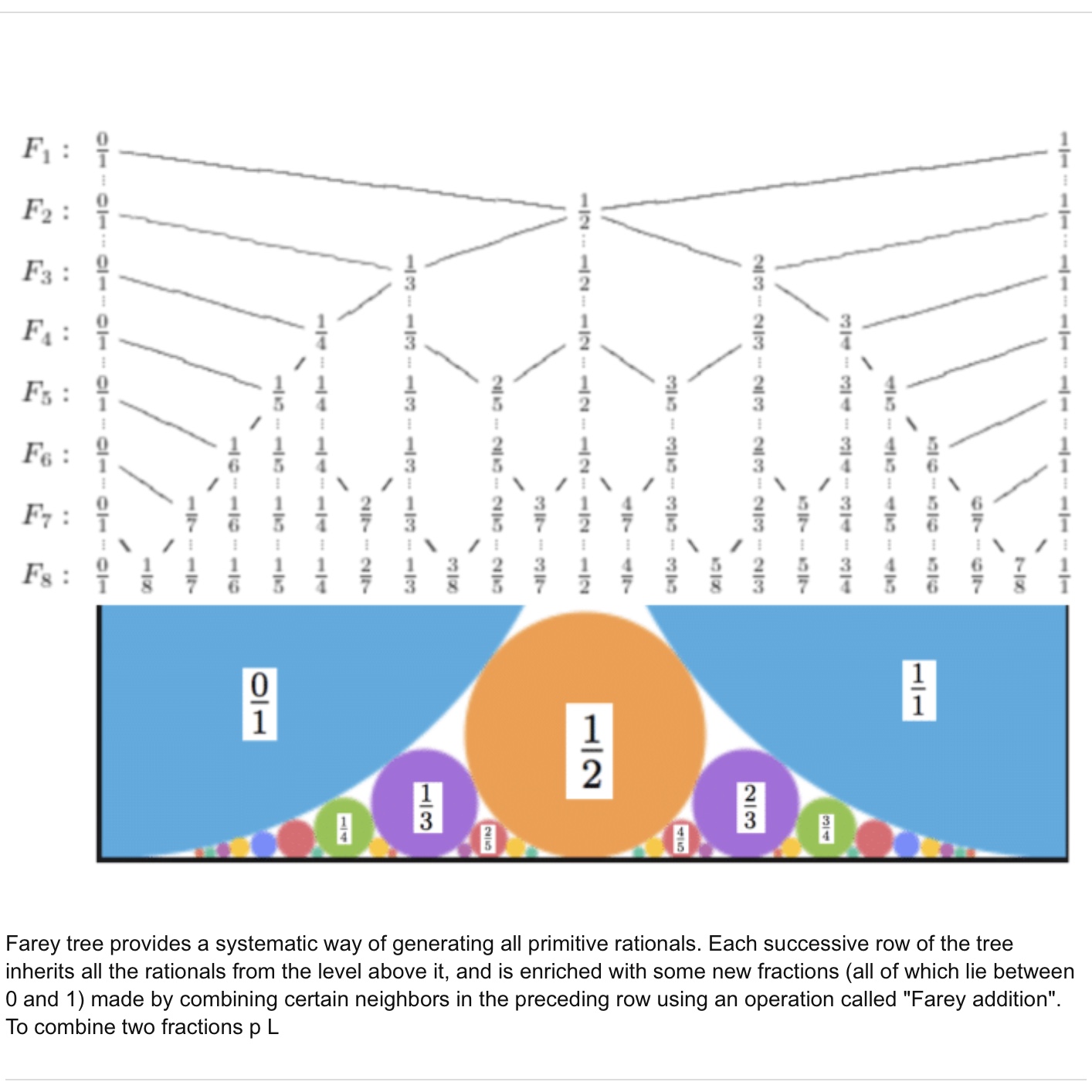
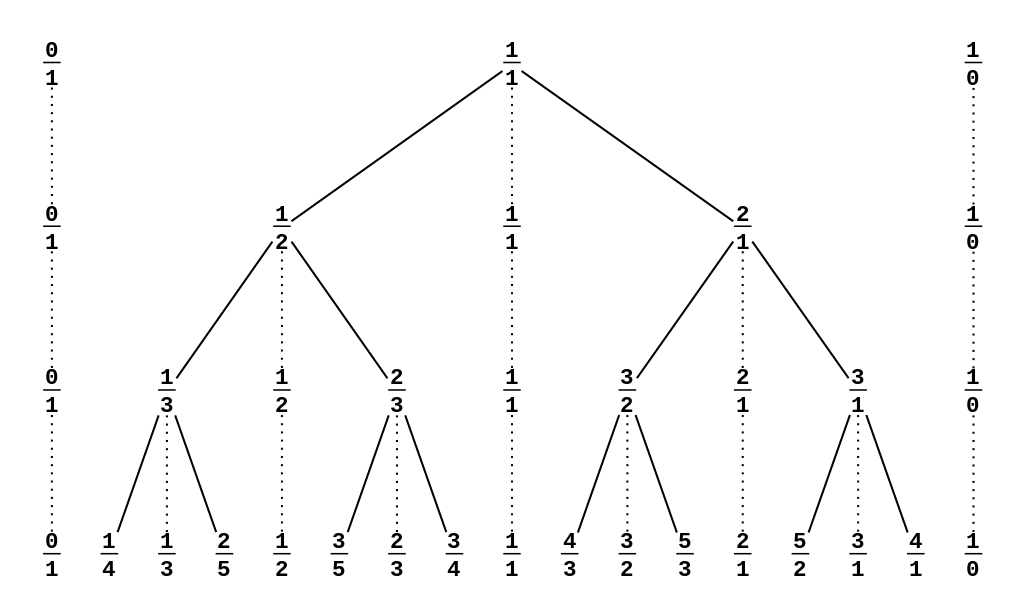
Every real number, rational and irrational, can be represented as a continued fraction. While normal fractions can only represent rational numbers, continued fractions are different - full of surprising patterns and relationships.
Not surprisingly, rational numbers produce finite continued fractions, while irrationals become infinite continued fractions.
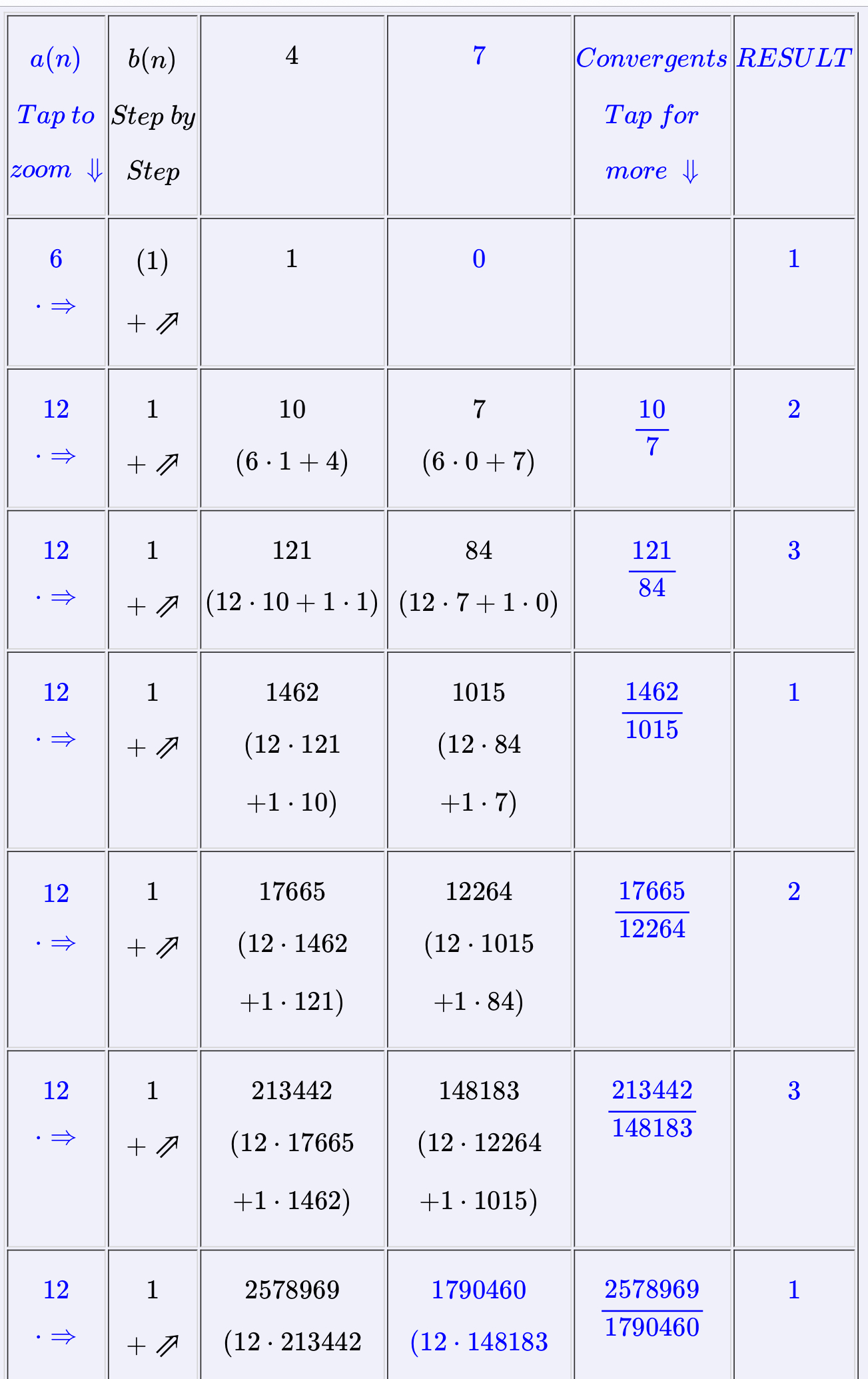
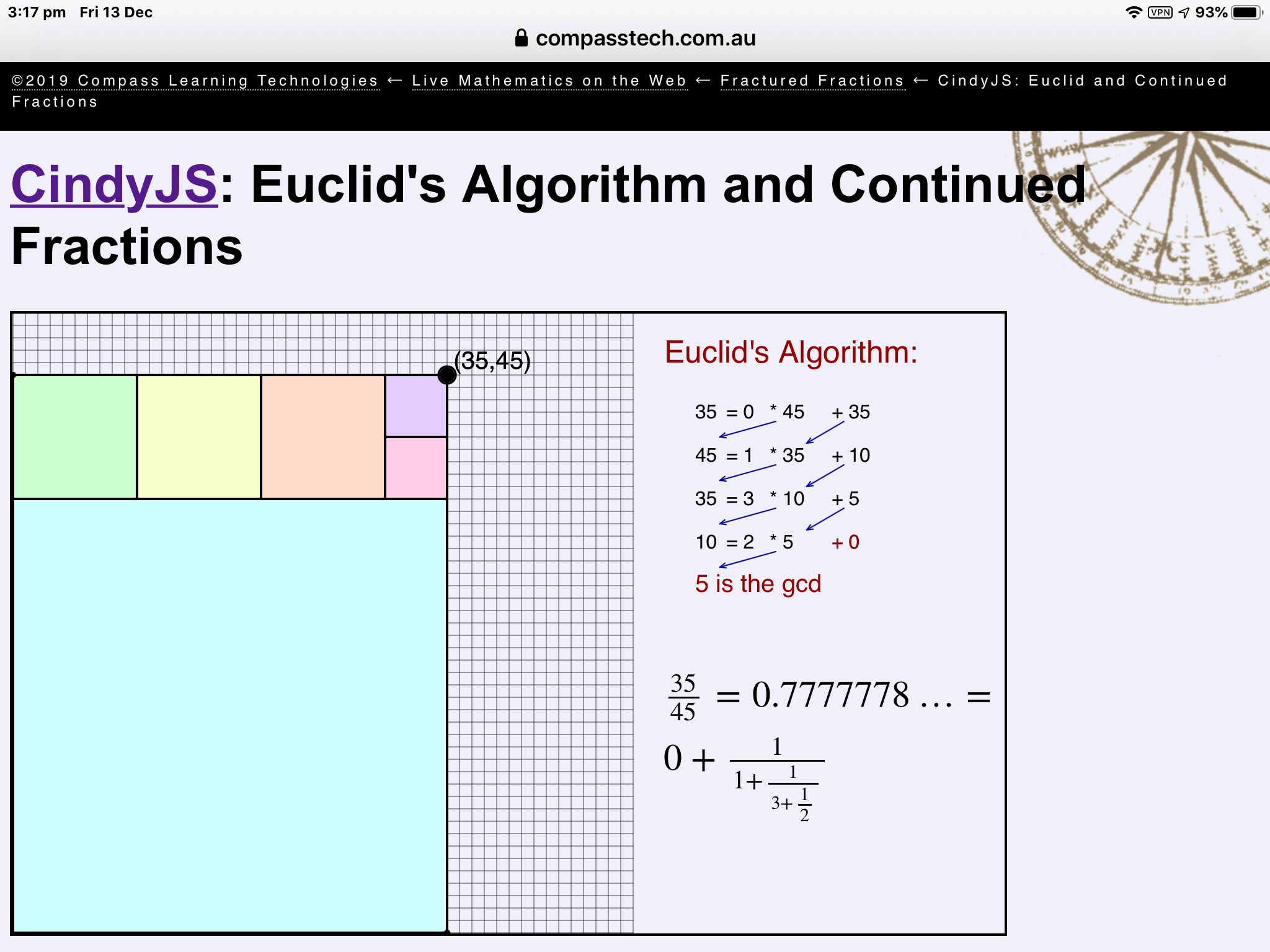
\[ \frac{10}{7}\]\[= 1+\frac{3}{7}\]\[= 1 + \frac{1}{\frac{7}{3}}\]\[= 1 + \cfrac{1}{2 + \cfrac{1}{3}} \]
Unlike irrational decimals, however, even irrational continued fractions can be predictable and are an ideal way to calculate approximate values - as accurately as you like!
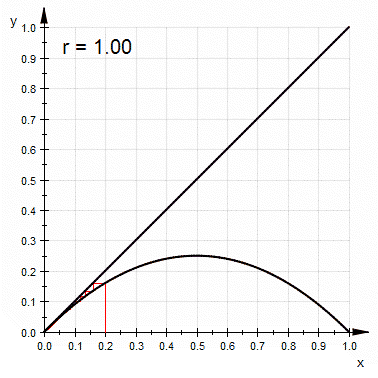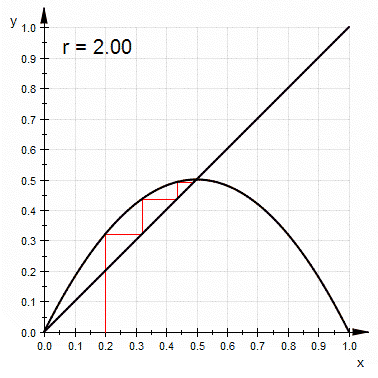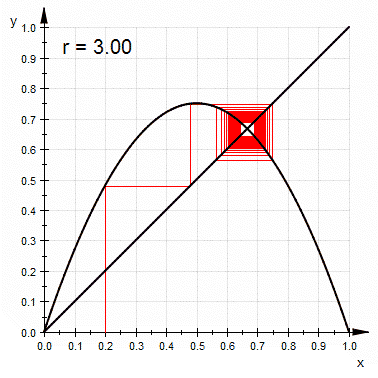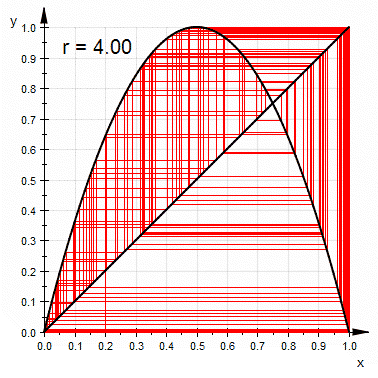
Continued fractions have many practical applications but one of the most important lies in their ability to offer VERY good approximations to irrational numbers - the more convergents, the better the approximation. Indeed, each convergent gives the Best Approximation of the First Kind (look that up!) AND if a large value turns up in the list, then cutting the continued fraction just before that large value gives an extremely accurate approximation!
Study the examples which follow and see what you notice.
Some Examples
Build Your Own Continued Fraction
Explore the Magic Table
Visualise Continued Fractions with GXWeb
About the MathBoxes...
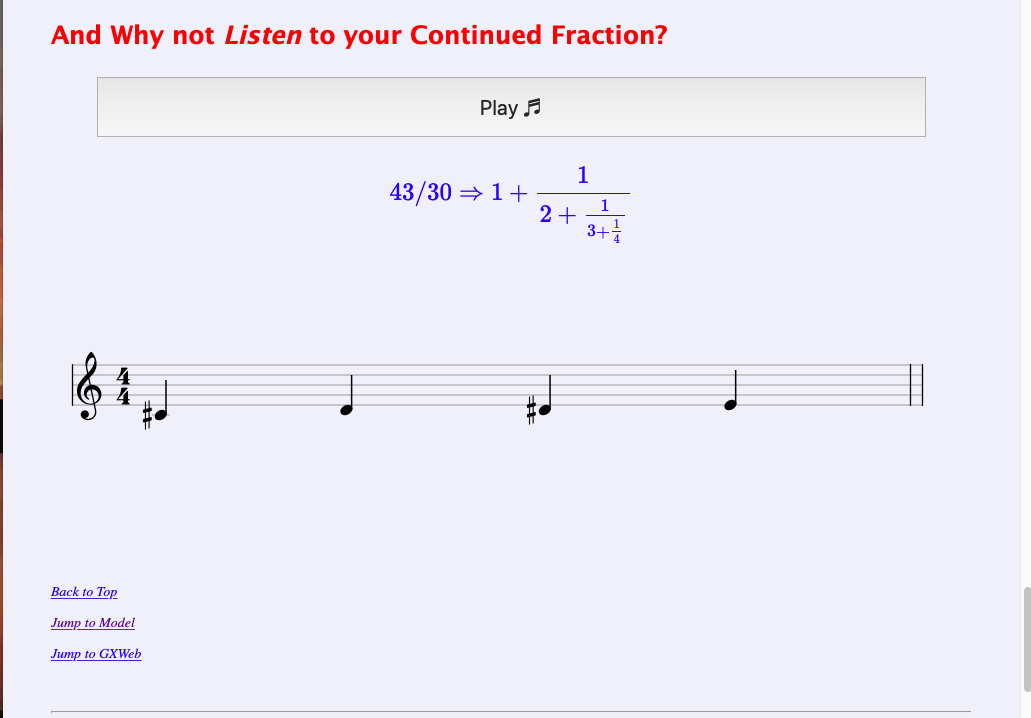
Use the Continued Fractions button or enter coordinates in the text boxes to explore (and even ♬ listen to!) your own continued fractions.