©2021 Compass Learning Technologies → Geometry Expressions Showcase → GXWeb Farey Numbers, Fraction Trees and Kissing Circles
GXWeb Farey Numbers, Fraction Trees and Kissing Circles
Saltire Software, home of Geometry Expressions and GXWeb
Download a Geometry Expressions Model for GXWeb Farey Numbers, Fraction Trees and Kissing Circles
Explore GXWeb Descartes Circle Theorem Model
Symbolic computations on this page use Nerdamer Symbolic JavaScript to complement the in-built CAS of GXWeb
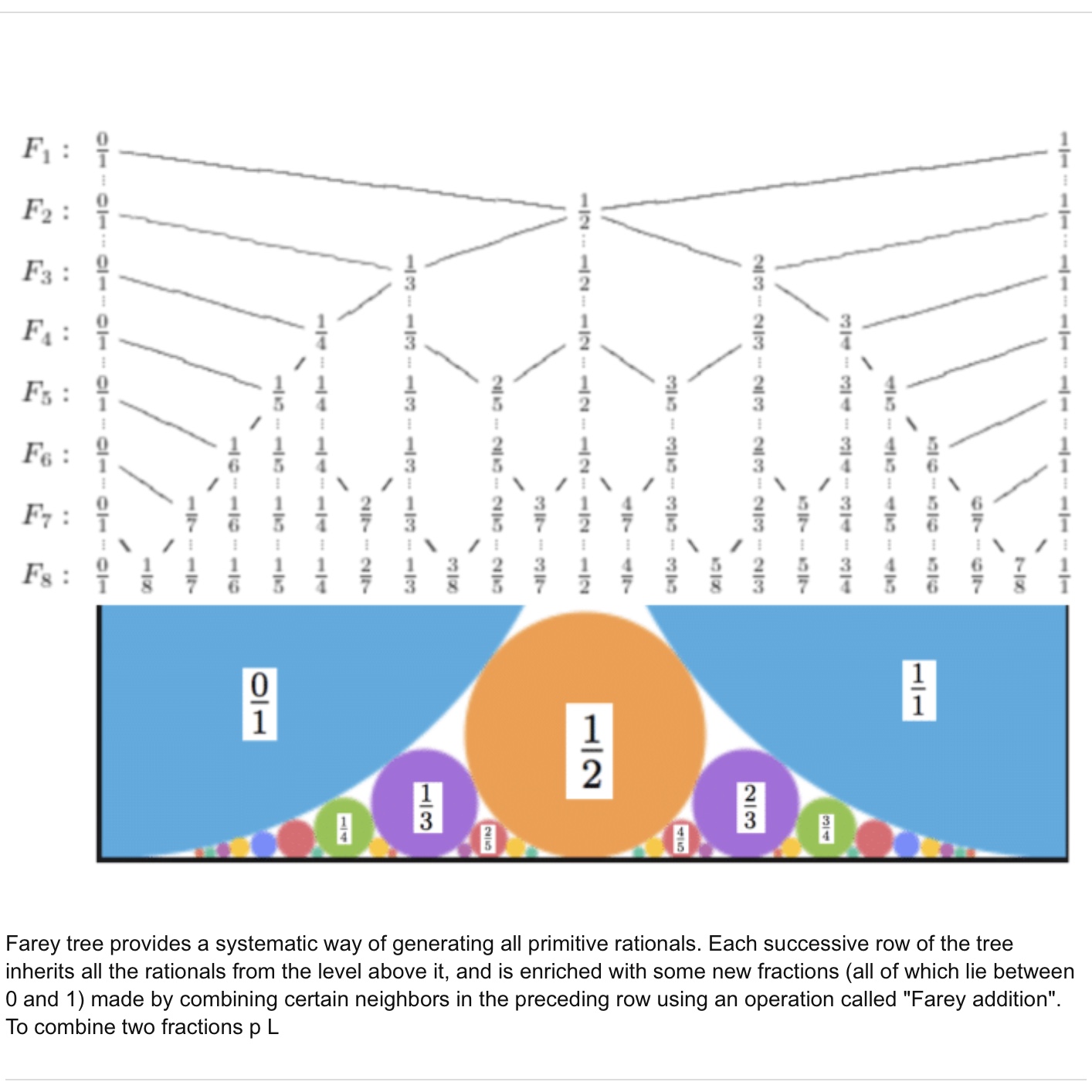
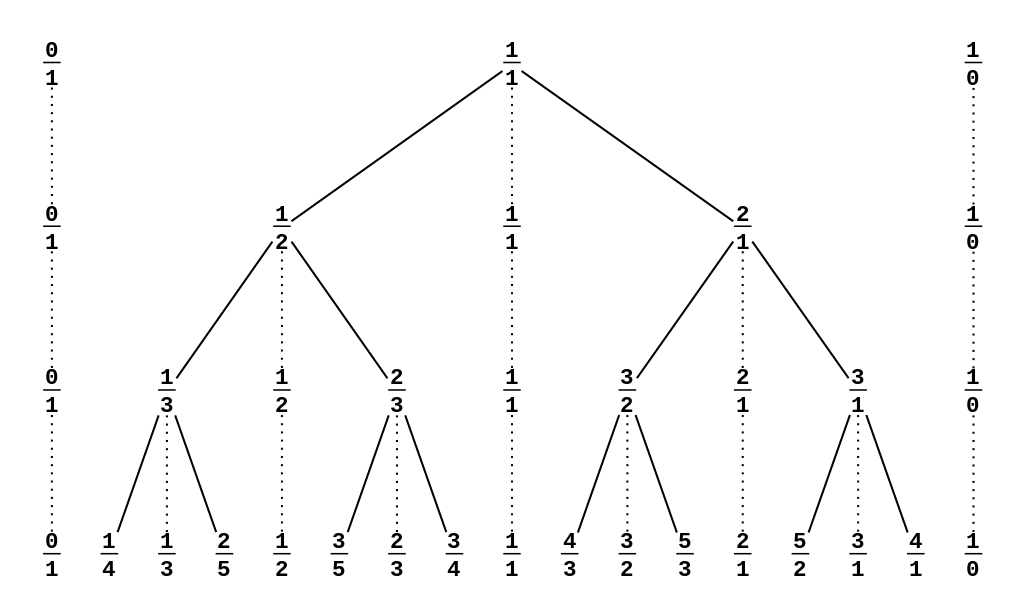
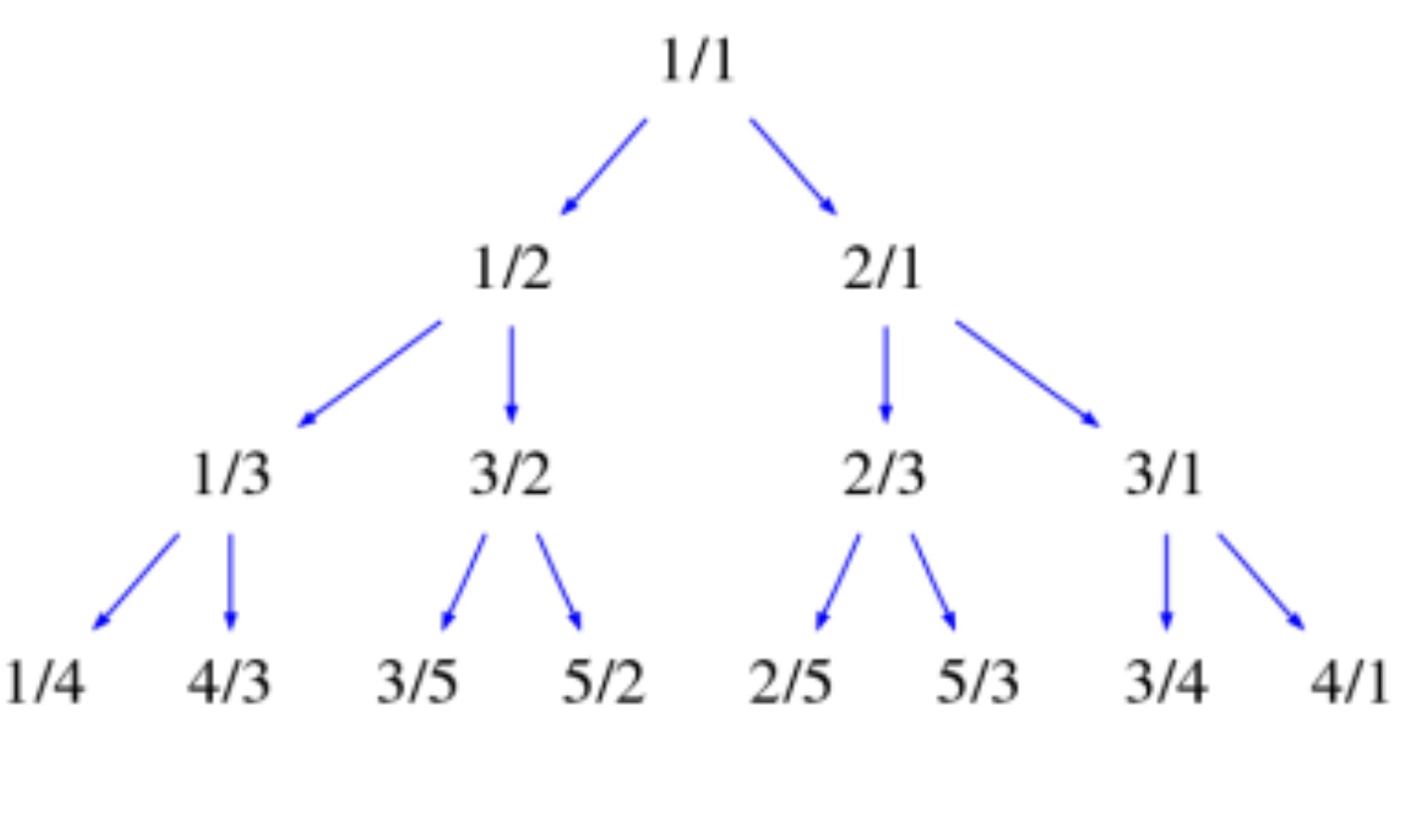
Sacred Geometry: Continued Fractions
Cut the Knot: The Stern-Brocot Tree
Calkin and Wilf (1999): Recounting the Rationals
Bates, B., Bunder, M. and Tognetti, K. (2010): Locating Terms in the Stern–Brocot Tree
Bates, B., Bunder, M. and Tognetti, K. (2010): Linking the Calkin-Wilf and Stern-Brocot trees
Bruce Bates (2014): The Stern-Brocot Continued Fraction
Ben Gobler: Listing the Rationals Using Continued Fractions (YouTube 15:09)
Ben Gobler: Listing the Rationals Using Continued Fractions 2 (YouTube 9:33)
Ben Gobler: Listing the Rationals Using Continued Fractions 3 (YouTube 13:42)
Climbing Around Fraction Trees with GXWeb (YouTube: 5:30)
Unpacking the Stern-Brocot Continued Fraction (YouTube: 7:34)
Climbing Around Fraction Trees and Unpacking the Stern-Brocot Continued Fraction
GXWeb Meaningful Algebra Collection
GXWeb Fractured Fractions Collection
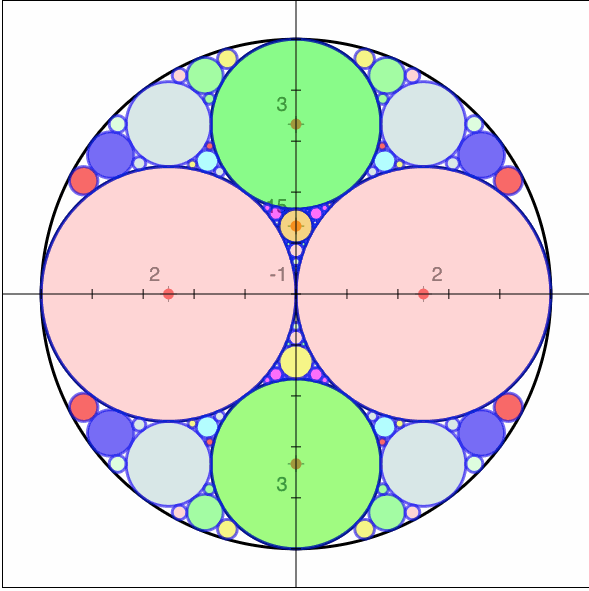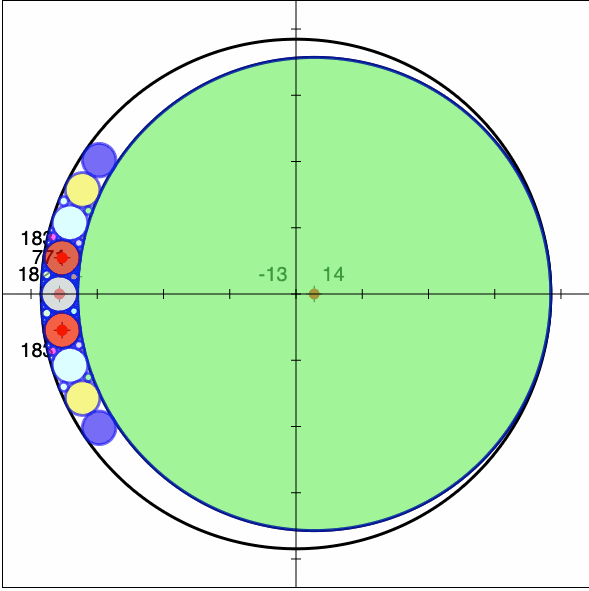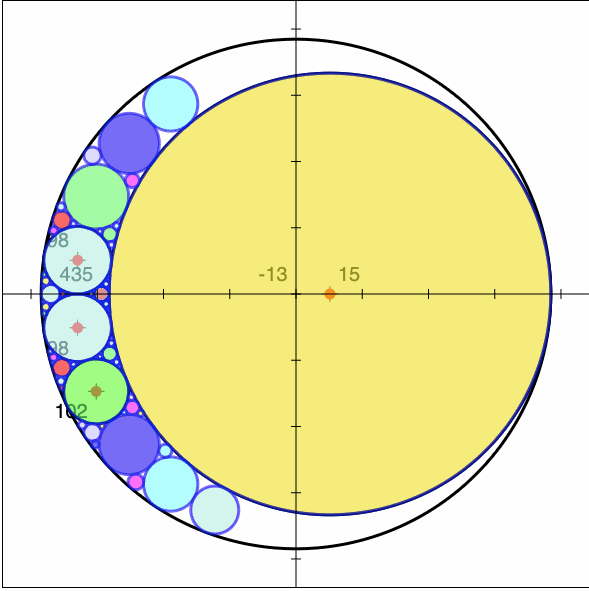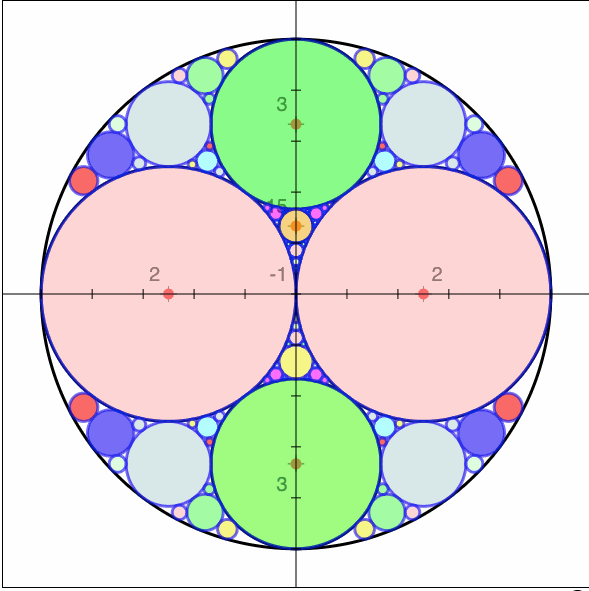
GXWeb Kissing Circles Collection
Take these ideas to the next level with the GXWeb Math Explorer
With thanks to Dr Keith Tognetti for pointing me in the direction of continued fractions many years ago and igniting in me a life-long passion, and to Dr Bruce Bates (both of the University of Wollongong) for revealing to us all the beautiful Stern-Brocot Continued Fraction. With colleague Dr Martin Bunder they have brought to light many wonderful and often surprising connections between binary trees and continued fractions.
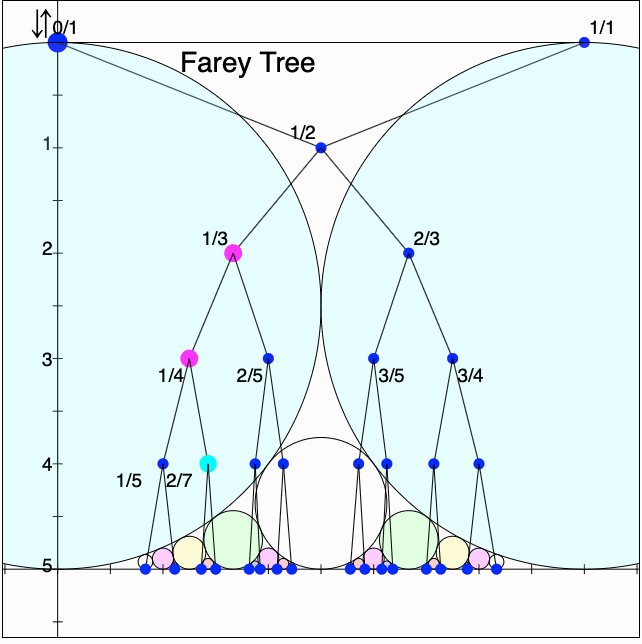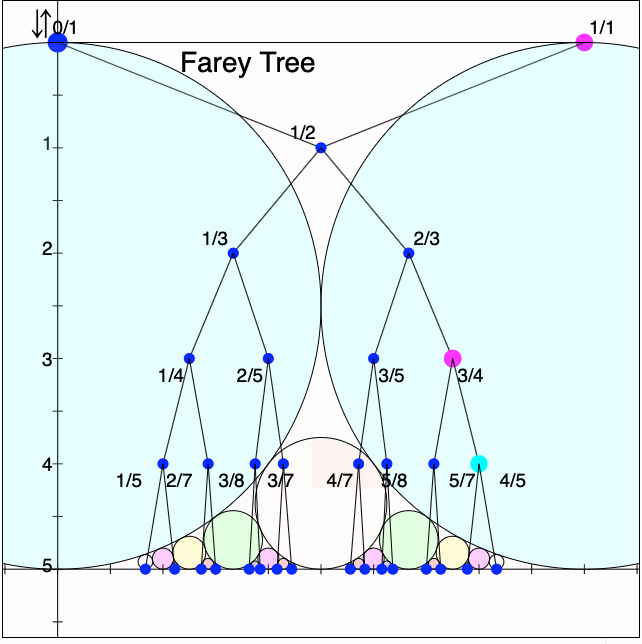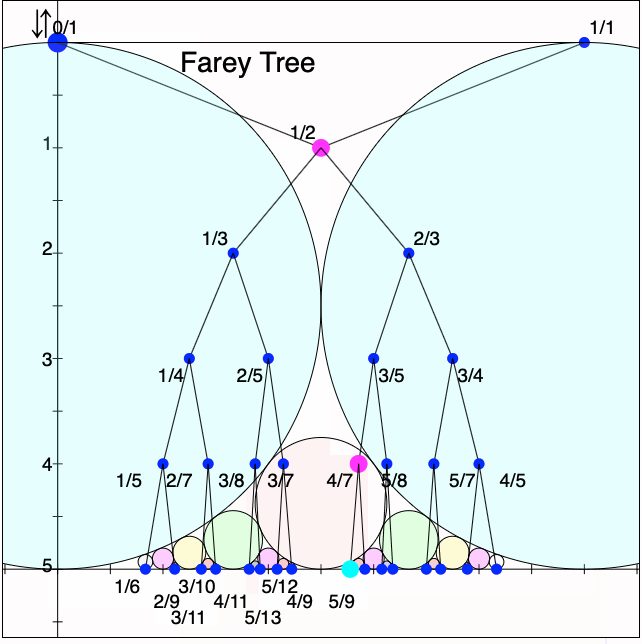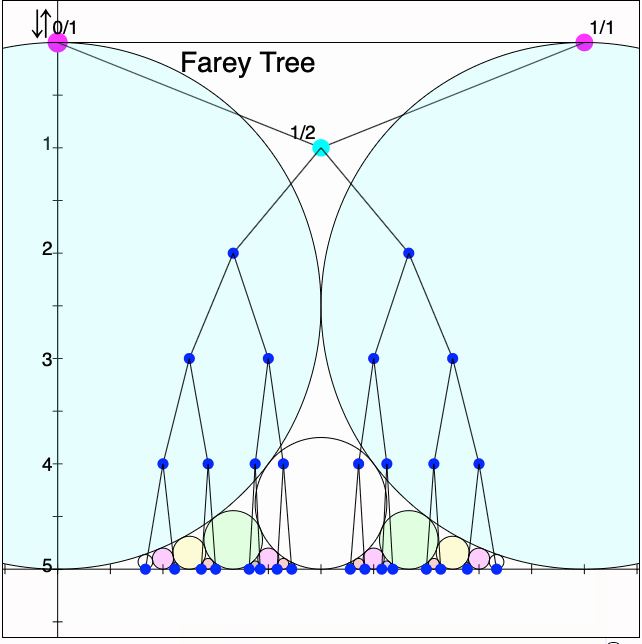
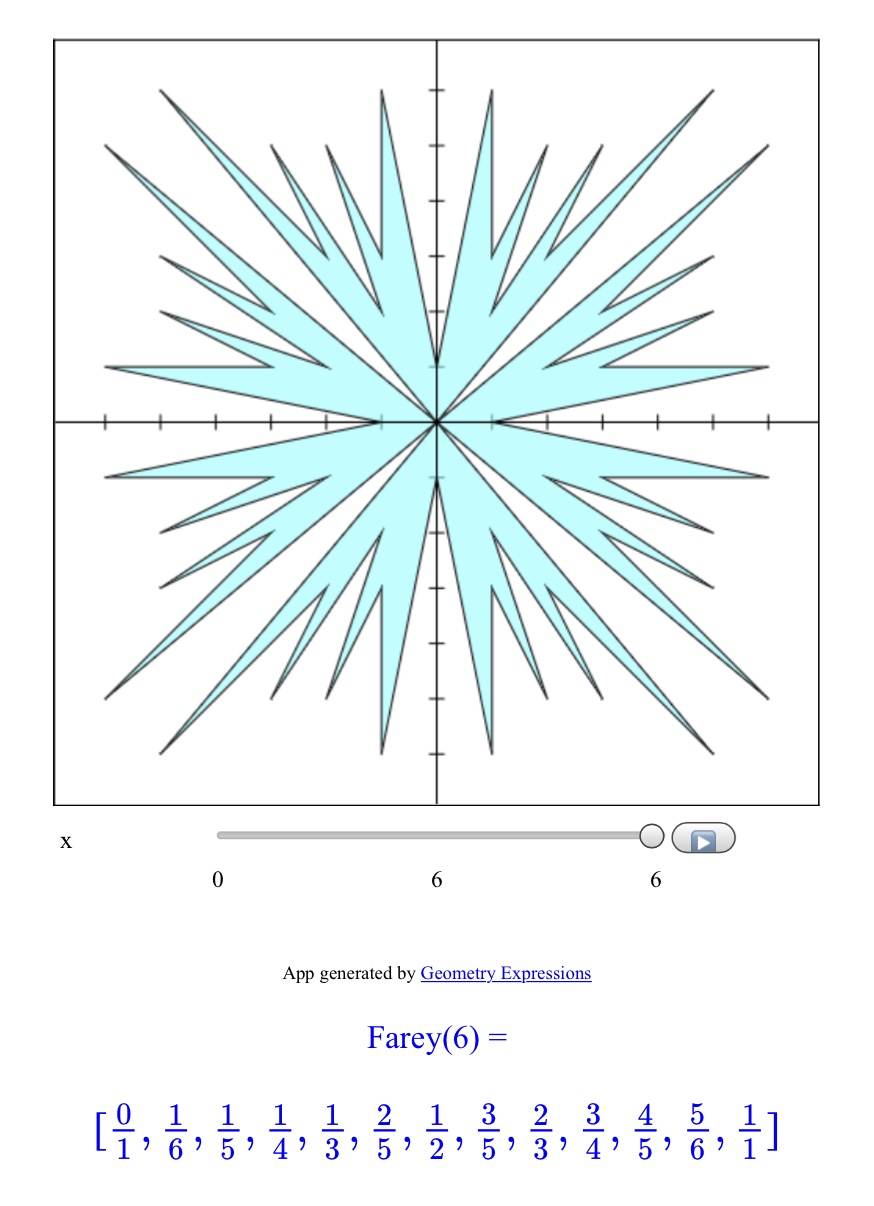
Suppose you wanted to make a list of all the rational numbers between 0 and 1.
How might you start?
One approach might be to begin with the denominators of the fractions: first, list those with denominator 1 - zero and 1: \(\frac{0}{1}, \frac{1}{1} \).
Next, add those with denominator 2: \(\frac{0}{1}, \frac{1}{2}, \frac{1}{1} \).
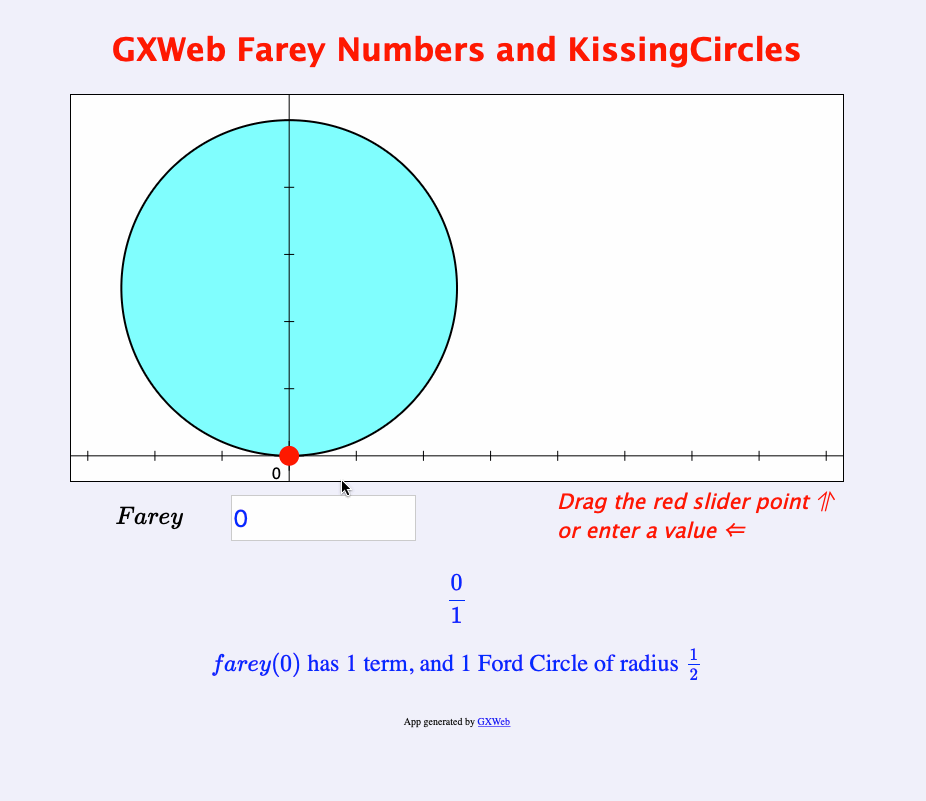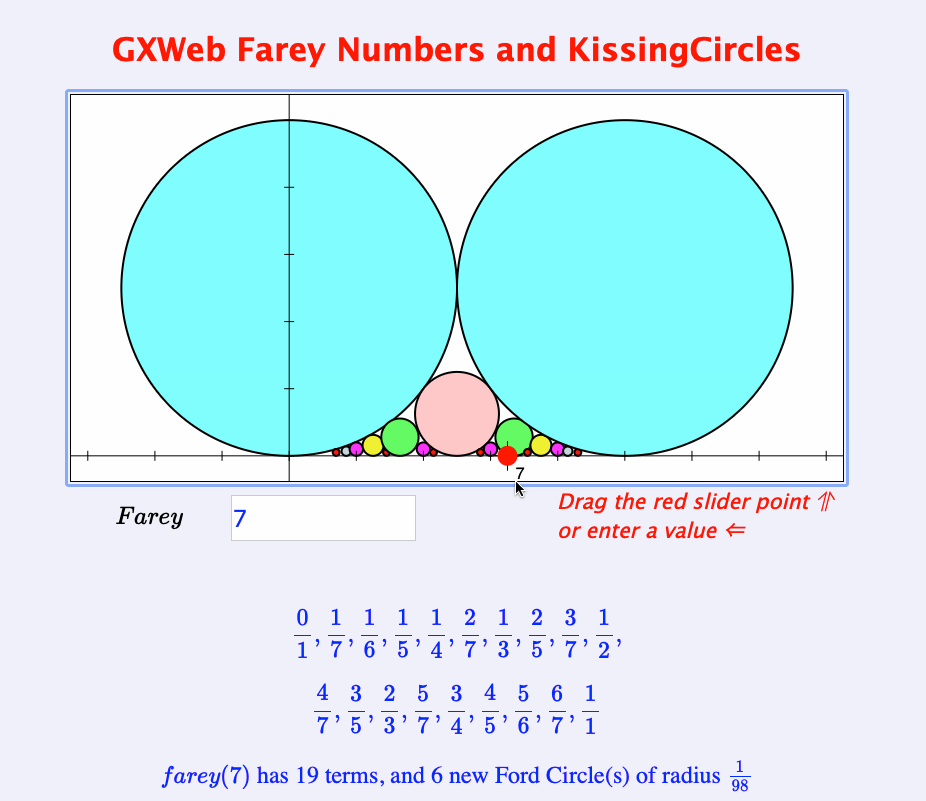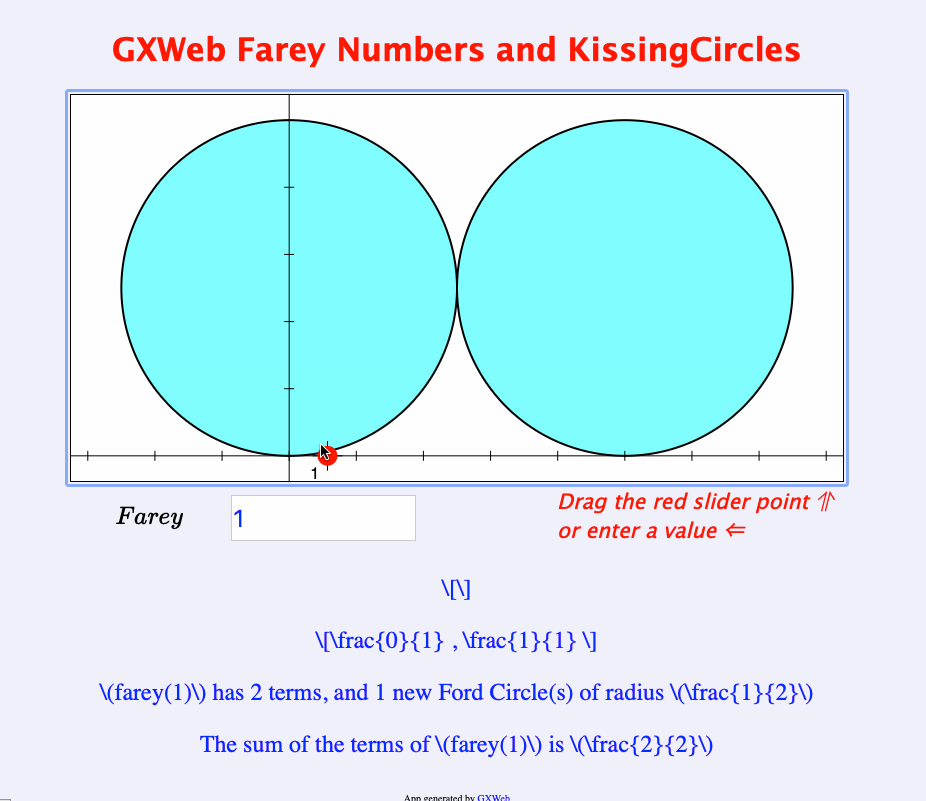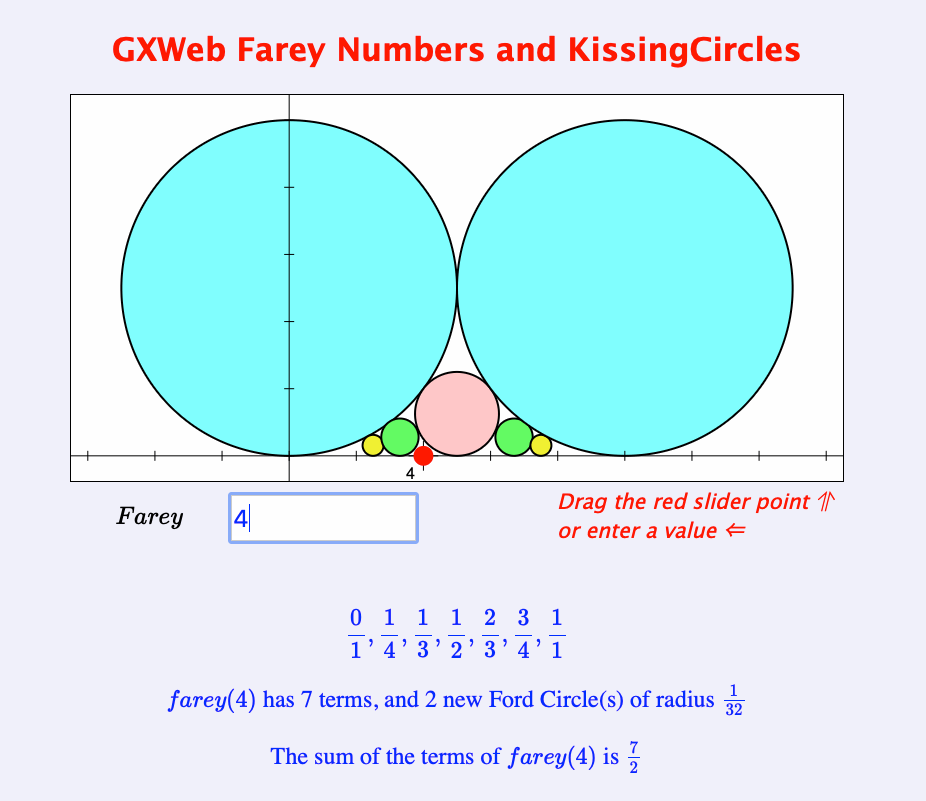
Add those with denominator 3 ( \(\frac{0}{1}, \frac{1}{3}, \frac{1}{2}, \frac{2}{3}, \frac{1}{1} \)) and you are on your way to the Farey Sequence! This last would be referred to as the Farey Sequence of Order 3.
So many patterns! So much to explore...
Introduction