©2023 Compass Learning Technologies ← GXWeb Showcase ← GXWeb Dragons, Folds and Fractions → Dragons, Folds and Fractions
GXWeb Dragons, Folds and Fractions
Saltire Software, home of Geometry Expressions and GXWeb
Symbolic computations on this page use Symbolic JavaScript to complement the in-built CAS of GXWeb
Explore with TI-Nspire? (TNSdragons.tns)
Explore GXWeb Dragon Curve Model
GXWeb Dragons, Folds and Fractions (YouTube)
Summed PaperFolding Sequences and Series (YouTube)
A Quick Paperfold (YouTube)
The Summed PaperFolding Sequence (YouTube)
Introduction: Here There Be Dragons
Take a piece of paper and fold it in half.
Fold again, in the same direction, and continue for a few folds.
If, after folding, you open the page out again, you will observe the paperfold sequence - a series of downward (▼ 1) and upward (▲ 0) folds (OR - if you were a turtle tracing out the path - left and right turns!) that may catch you by surprise!
If each fold is then opened out to create a right-angled corner, the resulting shape approaches the dragon curve fractal.
For example, a page folded 4 times will show the folds as
▼ ▼ ▲ ▼ ▼ ▲ ▲ ▼ ▼ ▼ ▲ ▲ ▼ ▲ ▲
[1,1,0,1,1,0,0,1,1,1,0,0,1,0,0]
OR even [R,R,L,R,R,L,L,R,R,R,L,L,R,L,L]
Twisting the Dragon's Tail
Our beautiful dragon curve is generally depicted with a turn angle of 90 degrees.
Can you imagine what our dragon curve would look like if the paper was bent at an angle other than 90 degrees?
Try turn angles like 45 degrees and 60 degrees. What do you think angles like 108 degrees (the Golden Dragon!), 120 degrees and 135 degrees might look like?
Importantly, is this potentially a way for us to peek inside the folded page in order to discover the order of the folds and the fractions hidden in these folds?
Finding the Fractions Hidden in the Folds
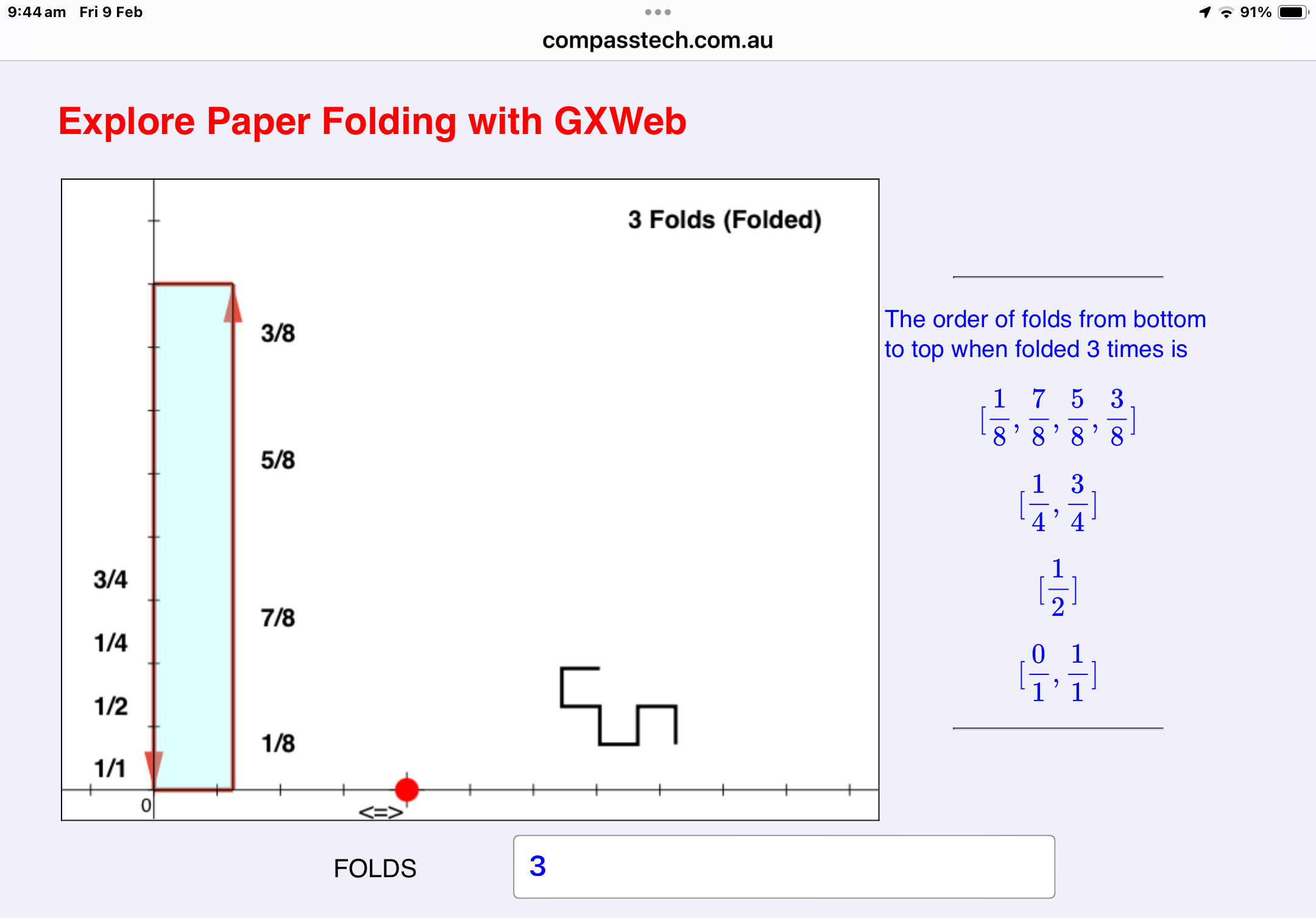
When a line joining 0 to 1 (or a rectangular piece of paper with [0;1] at its edge) is folded in half, right over left, the point 1/2 is at the fold. When the line is then folded in half again, right over left, the points 1/4 and 3/4 are at this second fold, in that order, from the outside of the fold to its interior.
Another fold and the sequence of points from the outside of the third fold to the inside becomes 1/8, 7/8, 5/8, 3/8.
Can you predict the order of the fourth fold? And the fifth?
Can you see how the fold fractions unfold in a series ofanti-clockwise spirals beginning with 0 and 1 (the edges of the page) and looping around the values at each level in successive order?
\[0,1\] \[\frac{1}{2}\] \[\frac{1}{4},\frac{3}{4}\] \[\frac{1}{8},\frac{7}{8},\frac{5}{8},\frac{3}{8}\]