©2020 Compass Learning Technologies → Live Mathematics on the Web → Geometry Expressions Assessment Showcase → Harmonic Mathematics
Harmonic Mathematics
Saltire Software, home of Geometry Expressions and GXWeb
Download a Geometry Expressions Model for Harmonic Mathematics
Try this activity with TI-Nspire™
Symbolic computations on this page use the GeoGebra CAS engine.
Introduction
Every once in a while in Mathematics we come across problems in which the numbers just do not seem to behave themselves. They appear simple enough at the outset, but we quickly discover that our mathematical intuition fails us, and the solution - if it comes at all - arrives as the result of a long and tortuous process.

In such cases, it is not uncommon to find that lurking behind the problem - often undiscovered - lies a fascinating and historically significant fellow called the harmonic mean. Certainly known to Pythagoras - to whom the four branches of Mathematics were arithmetike (number theory), logistike (calculation), geometrike (practical geometry) and armoniai (music) - the Harmonic Mean (2⋅a⋅ba+b) has been all but forgotten in modern Mathematics, overshadowed by its more famous relatives, the Arithmetic (a+b2) and Geometric (√(a⋅b)) means. Its neglect is sad, since it not only touches upon many elegant and beautiful elements in arithmetic, algebra and geometry, but it also has some interesting applications which further justify its study.
Try building your own model for this problem using GXweb and use it to explore both the geometry and the algebra involved.
What Do You Mean?
It is worthwhile first to consider the nature of Means. The growth in the study of Statistics in recent times has led to the common misconception that there is only the Mean. It is usually with some satisfaction that teachers of Senior Mathematics point out to their students that, in addition to the Arithmetic Mean there is also a Geometric Mean, But, of course, there are many, many means.
In arithmetic, a mean is usually thought of as a mathematical process which places a number somewhere in relation to two given numbers. The Arithmetic Mean, of course, is that number exactly halfway between two numbers, given by the formula AM(a,b)=a+b2
The Geometric Mean, on the other hand is always less than the Arithmetic Mean, and is produced as follows GM(a,b)=√a×b
Any mathematical process which takes two numbers and uses these to produce a third can be thought of as a mean provided it passes one test. A good mean when asked to operate upon the same number twice should always produce that number as the answer. Thus, from the formulae above, AM(a, a) = GM(a, a) = a.
We note, too, that the original numbers and their mean form a sequence or progression. Thus, if y is the arithmetic mean of x and z then x, y, z are said to be in arithmetic progression. Similarly, the geometric mean and its two source numbers form a geometric progression.
The Harmonic Mean may be defined in the following way. Three numbers, a, b and c are said to be in harmonic progression if their reciprocals are in Arithmetic Progression. The actual formula for the Harmonic Mean may then be derived by either using the formula above for the Arithmetic Mean, where 1b=12⋅(1a+1c)
or by using the familiar property of arithmetic progressions concerning the constant difference between terms, such that 1b−1a=1c−1b
Either way, it is a useful manipulative exercise for students to isolate c and produce the formula HM(a,b)=2⋅a⋅ba+b
(You should also check that this formula passes the test for means!)
Interested to learn more?
Calculate the Means
NOTE: You can use our mean calculator as an approximation tool for real numbers. For example, to calculate the best approximation your device can offer for a real number n, say, √2, begin by pressing the reset button, then enter sqrt(2) for a, and your guess (say 1.4) for b. Now simply repeat the process, but do not change the set values - just press OK to accept these. Then the geometric mean value of the result will be the best approximation for your original real number!
What just happened? On the second round, a takes the value of your approximation (call it a1 - in this case, 1.4), while b takes the value of n2a1. Can you see why this gives the geometric mean as the best approximation for n?
Note also that the values for arithmetic mean and harmonic mean very closely sandwich the actual value for the real number you are approximating - they too are excellent approximations!
Interested to learn more?
Harmony, Music and Mathematics
Music is the Mathematics of one who does not know that he is counting. Gottfried Wilhelm Leibniz (1646-1716)
When Pythagoras discovered that the major musical tones could be produced by shortening a string by simple whole number ratios, he had little doubt that eventually all of nature could be described and explained through the use of numbers - hence the motto of the secret society of which he was the founder and inspiration: All is number. He was excited to discover that the octave was produced by the ratio 2 : 1, the major fourth 3 : 2 (the arithmetic mean of 1 and 2), and the major fifth 4 : 3 (the harmonic mean of 1 and 2). The musical scale which Pythagoras deduced from these ratios was quite different from our modern scale (which is based upon a geometric sequence with common ratio 12√2. He certainly applied his musical scale, among other things, to predict the relative positions of the sun and the planets from the earth (the harmony of the spheres), and to his theories of healing and medicine.
Pythagoras used the word harmony in a different sense than that which we use today, in which we describe a pleasant sounding combination of notes. To the Pythagoreans, armoniai described a well-ordered sequence of notes - a pattern or scale that was pleasing to the ear. Noone knows what this original scale was, but it seems likely that it was based upon the calculation which he himself named the harmonic mean.
Consider, for instance, the following ratios as one way of representing the fourth, fifth and octave notes of the scale:
C 1 : 1 F 4 : 3 G 3 : 2 C' 2 : 1 Then, by taking the harmonic mean of C and G it is possible to calculate a ratio for E; HM(C, E ) produces D, F and C' produce A, and A and C' can be used to find B. In this way, a scale emerges:
Note Harmonic
RatioHarmonic
DecimalWell-tempered
ScaleC 1 : 1 1.0 1.0 D 12 : 11 1.091 1.122 E 6 : 5 1.2 1.26 F 4 : 3 1.333 1.335 G 3 : 2 1.5 1.498 A 8 : 5 1.6 1.682 B 16 : 9 1.778 1.888 C' 2 : 1 2.0 2.0 Can you determine how each of the harmonic ratios was derived?
While this scale produces tones surprisingly close to the modern well-tempered scale, there are inconsistencies. (Using the Harmonic Mean method, for example, offers several options for computing parts of the scale - notes like A and B, in particular might be calculated in different ways). To go beyond a single octave, one would double each value to produce the next octave, and double each of those, in turn. The modern well-tempered scale is much more consistent across the range of notes (although might a scale based upon an arithmetic series also be consistent?)
Compare the sound of our modern (well-tempered) scale (based upon a geometric sequence) to that of one based upon the harmonic series of Pythagoras...
Choose a wave form for your tone...
Compare the sound of our modern (well-tempered) scale (based upon a geometric sequence) to that of one based upon the harmonic series of Pythagoras...
Choose a song...
Timing: Key Signature:
In the sense that the Pythagoreans were attempting to use Mathematics to describe and explain a universe which they did not understand, Mathematics itself could well be defined as a search for harmony - a search for patterns and relationships which will impose an order, an armoniai, upon an otherwise chaotic world.
Pictures of Harmony
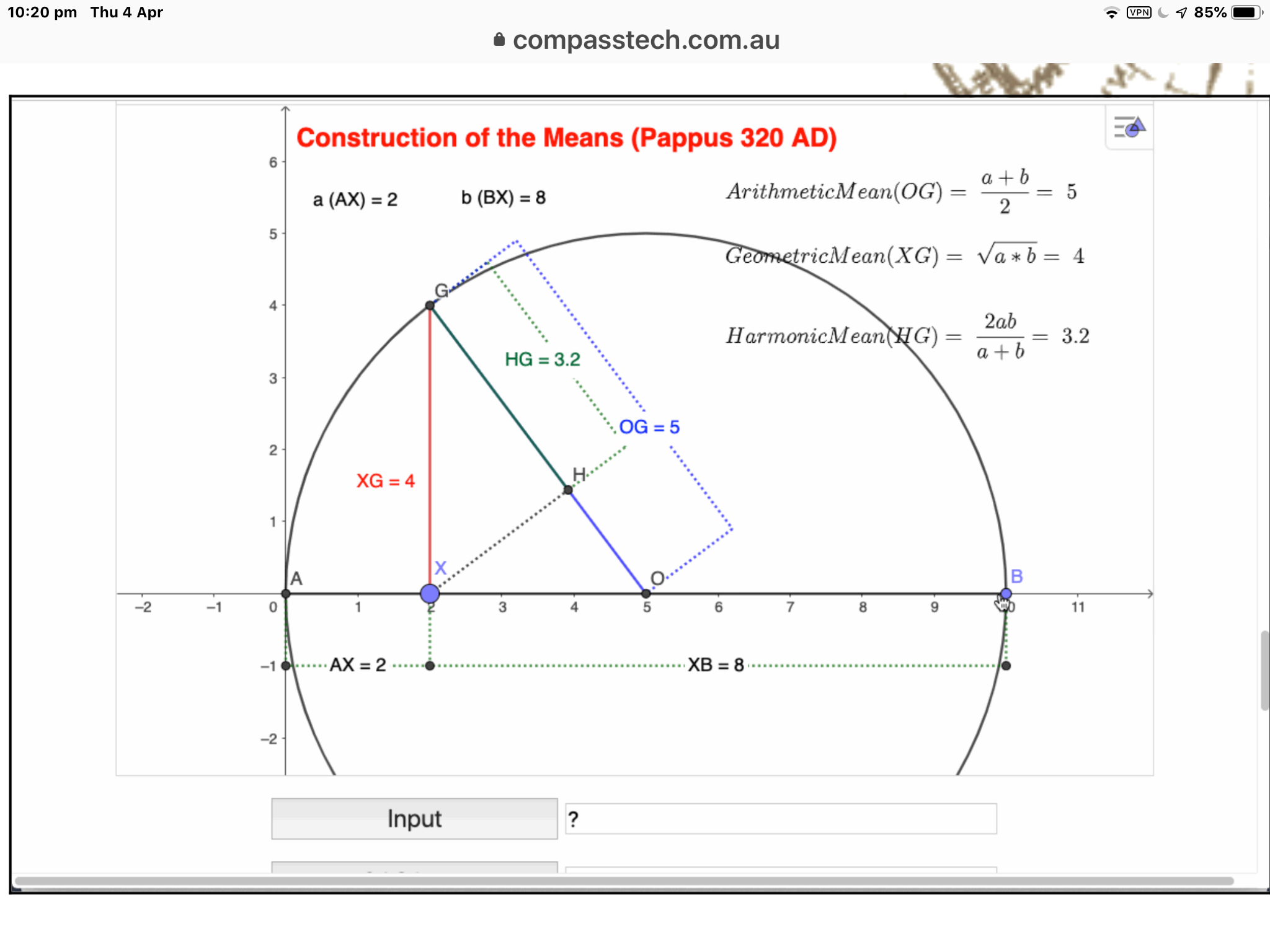
There are several lovely geometric constructions of these three means, but few are as elegant or convincing as that attributed to Pappus of Alexandria, around 320 AD, as shown in the Geometry Expression model which follows.
This construction clearly demonstrates a most important property of the means, that for all distinct (non-equal values): AM > GM > HM
Can you see how this is proven geometrically below? Drag the point X in the live model that follows to explore these relationships further.
Interested to learn more?
And for an entirely different picture of harmony...⇒