1. Drag the point P to find the height value for which the area of the triangle is greatest.
2. Build an algebraic model for the area of the triangle by defining the following functions: height(x) =
3. hypot(x) =
4. base(x) =
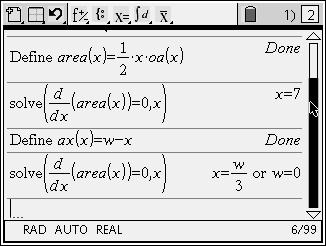
5. area(x) =
6. Using Calculus build the derivative function for area(x) and use this to find the exact value of the height for which the area of the triangle is greatest: myderiv(x) =
7. myderiv(x) = 0 when x =
How difficult did you find this activity? Please assign a rating value from 1 (very easy) to 5 (extremely difficult). Comments? Suggestions? What did you learn from this activity?
My name:
My class:
Teacher Email:
Peter Fox and Steve Arnold. Created with GeoGebra
This problem may also be modelled using CabriJr on the TI-83/84 series calculator. Click on the graphic below to download the file and try it yourself!
Try having students work in pairs: one generating data points using the CabriJr model and the partner entering these into lists to plot and then to help build their algebraic model!
And just wait until you try this with TI-nspire CAS!
This dynamic figure was created using the latest version of the free GXWeb from Saltire Software, as shown below.

©2019 Compass Learning Technologies ← Live Mathematics on the Web ← GeoGebra Showcase ← The Paper Fold Problem