©2020 Compass Learning Technologies → Live Mathematics on the Web → GXWeb Curve Construction Collection → GXWeb Strophoid
GXWeb Constructing the Strophoid (1)
Saltire Software, home of Geometry Expressions and GXWeb
GXWeb Curve Construction Collection (like this one!)
About the Strophoid (from Wolfram MathWorld)
A strophoid is a curve generated from a given curve C and points A (the fixed point) and O (the pole). In the special case where C is a line, A lies on C, and O is not on C, then the curve is called an oblique strophoid. If, in addition, OA is perpendicular to C then the curve is called a right strophoid, or simply strophoid by some authors. The right strophoid is also called the logocyclic curve or foliate.
| \(a\) | |||||
| 0 | 0 | 5 | |||
| \(\theta\) | |||||
| -6.2832 | 0 | 6.2832 | |||
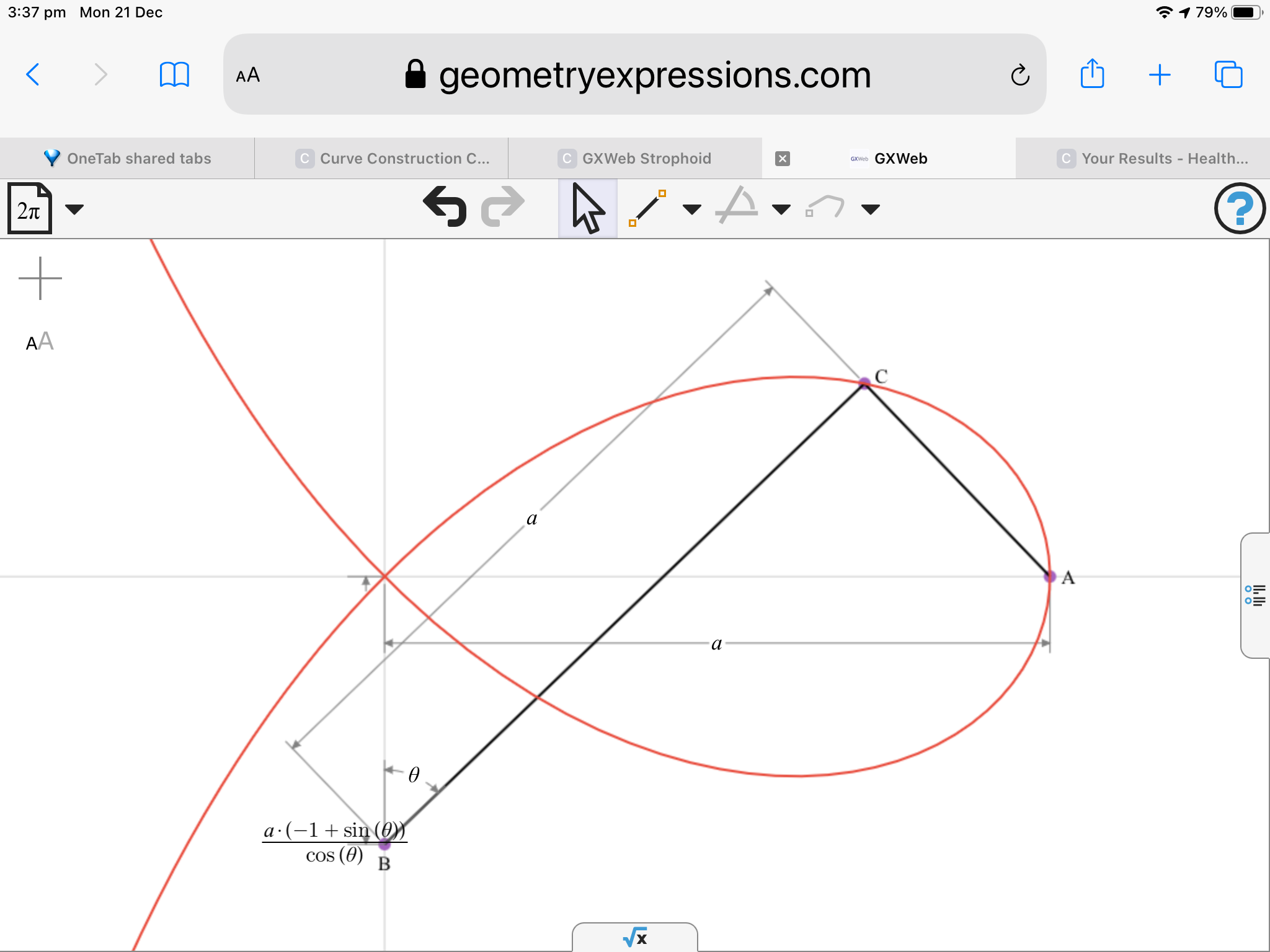 | The right strophoid curve is the locus of point C where \(a = Length(OA) = Length(BC)\) \(\angle OBC = \theta\) and \(angle(BCA) = \frac{\pi}{2}\). |
Cartesian equation: \[y^2=x^2 \cdot \frac{a-x}{a+x}\]
Parametric equation: \[x(t)=a^2 \cdot \frac{a^2-t^2}{a^2+t^2}\] \[y(t)=t \cdot \frac{t^2-a^2}{t^2+a^2}\]
Try this yourself with the tools below...
and then try out a simpler construction!
Construct your own Model with GXWeb
WolframAlpha: CAS+
The powerful Wolfram Alpha online CAS engine will answer almost anything you care to ask - within reason! From the continued fraction of pi to Solve x^2=x+1 to the population of Australia!
Behind the Scenes
©2020 Compass Learning Technologies ← Live Mathematics on the Web ← GXWeb Curve Construction Collection ← GXWeb Strophoid (1)