©2020 Compass Learning Technologies → Live Mathematics on the Web → GXWeb Curve Construction Collection → GXWeb Hyperbola
GXWeb Hyperbola
Saltire Software, home of Geometry Expressions and GXWeb
GXWeb Curve Construction Collection (like this one!)
About the Hyperbola (from Wolfram MathWorld)
The Hyperbola is a plane curve for which the difference of the distances from two fixed points (the foci A and D) separated by a distance is a positive constant.
The ellipse and the hyperbola are closely related conics - can you see the values for a and f in this model for which it switches from hyperbola to ellipse and back again? Use the model to explore and study the cartesian equation below.
| 0 | 5 | 10 | |||
| 0 | 3 | 10 | |||
| 0 | 1.32 | 1.57 | |||
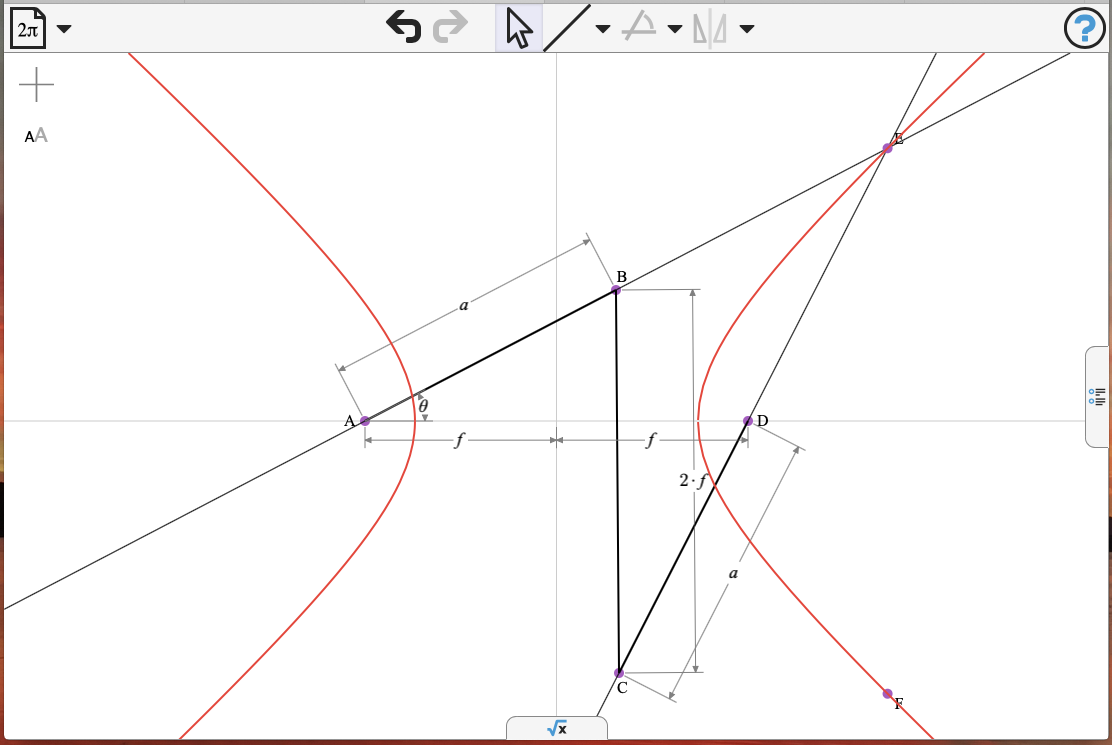 | The Hyperbola constructed here is the locus of points E and F where
and . |
Cartesian equation:
Try this yourself with the tools below...
Construct your own Model with GXWeb
WolframAlpha: CAS+
The powerful Wolfram Alpha online CAS engine will answer almost anything you care to ask - within reason! From the continued fraction of pi to Solve x^2=x+1 to the population of Australia!
Behind the Scenes
©2020 Compass Learning Technologies ← Live Mathematics on the Web ← GXWeb Curve Construction Collection ← GXWeb Hyperbola